Although there are extensive tables available for the pK_a of weak acids, you might be dealing with an unknown acid or a known acid at an unlisted temperature. You could then use a procedure like this to determine the K_a and pK_a. The pH of a 0.010 m aqueous solution of a certain carboxylic acid is 2.95. What are its K_a and pK_a?

ANTICIPATE All carboxylic acids are weak acids; therefore, expect K_a ≪ 1.

PLAN Calculate the hydronium ion concentration from the pH and then calculate the value of K_a from the equilibrium concentration of the acid and the hydronium ion concentration.

What should you assume? As in Example 6D.1, because pH

Example 6D.1

Acetic acid is a weak acid commonly found in both laboratory and household, but to what extent have its molecules actually been deprotonated? Calculate the pH and percentage deprotonation of CH₃COOH molecules in 0.080 m CH₃COOH(aq), given that K_a for acetic acid is 1.8 * 10^–5.

ANTICIPATE Because the solution is that of an acid, expect pH

PLAN Following the procedure in Toolbox 6D.1, write the proton transfer equilibrium and construct the equilibrium table with concentrations in moles per liter.

What should you assume? You can make two assumptions, but they need to be verified at the end of the calculation.

(1) Deprotonation is so slight that the equilibrium concentration of the acid is approximately the same as its initial concentration.

(2) The autoprotolysis of water does not contribute significantly to the pH.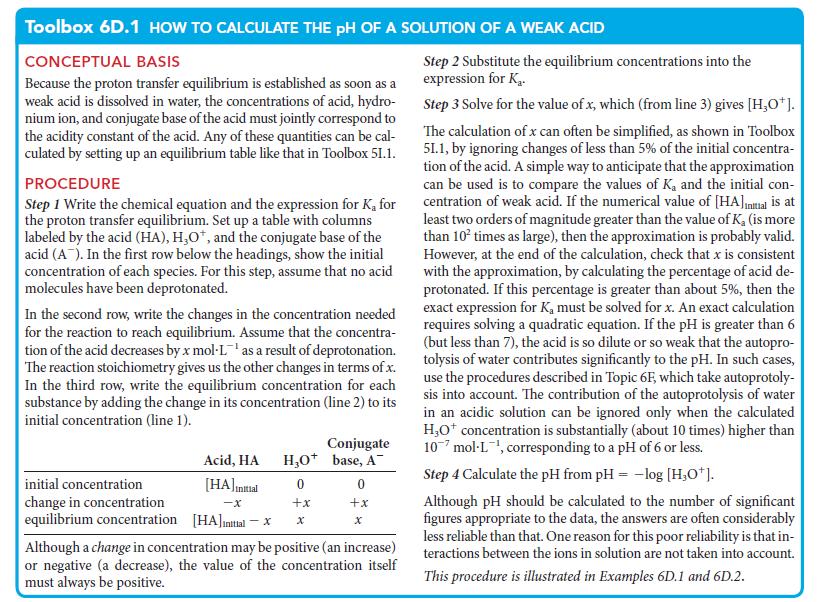

Transcribed Image Text:

Toolbox 6D.1 HOW TO CALCULATE THE PH OF A SOLUTION OF A WEAK ACID CONCEPTUAL BASIS Because the proton transfer equilibrium is established as soon as a weak acid is dissolved in water, the concentrations of acid, hydro- nium ion, and conjugate base of the acid must jointly correspond to the acidity constant of the acid. Any of these quantities can be cal- culated by setting up an equilibrium table like that in Toolbox 51.1. PROCEDURE Step 1 Write the chemical equation and the expression for K, for the proton transfer equilibrium. Set up a table with columns labeled by the acid (HA), H₂O*, and the conjugate base of the acid (A). In the first row below the headings, show the initial concentration of each species. For this step, assume that no acid molecules have been deprotonated. In the second row, write the changes in the concentration needed for the reaction to reach equilibrium. Assume that the concentra- tion of the acid decreases by x mol-L¹ as a result of deprotonation. The reaction stoichiometry gives us the other changes in terms of x. In the third row, write the equilibrium concentration for each substance by adding the change in its concentration (line 2) to its initial concentration (line 1). initial concentration change in concentration equilibrium concentration Acid, HA [HA] initial -X [HA]initial - x H₂O* 0 +x X Conjugate base, A™ 0 +x X Although a change in concentration may be positive (an increase) or negative (a decrease), the value of the concentration itself must always be positive. Step 2 Substitute the equilibrium concentrations into the expression for K₂. Step 3 Solve for the value of x, which (from line 3) gives [H₂0*]. The calculation of x can often be simplified, as shown in Toolbox 51.1, by ignoring changes of less than 5% of the initial concentra- tion of the acid. A simple way to anticipate that the approximation can be used is to compare the values of K, and the initial con- centration of weak acid. If the numerical value of [HA]Initial is at least two orders of magnitude greater than the value of K. (is more than 10² times as large), then the approximation is probably valid. However, at the end of the calculation, check that x is consistent with the approximation, by calculating the percentage of acid de- protonated. If this percentage is greater than about 5%, then the exact expression for K, must be solved for x. An exact calculation requires solving a quadratic equation. If the pH is greater than 6 (but less than 7), the acid is so dilute or so weak that the autopro- tolysis of water contributes significantly to the pH. In such cases, use the procedures described in Topic 6F, which take autoprotoly- sis into account. The contribution of the autoprotolysis of water in an acidic solution can be ignored only when the calculated H₂O* concentration is substantially (about 10 times) higher than 10 mol-L¹, corresponding to a pH of 6 or less. Step 4 Calculate the pH from pH = -log [H,0¹]. Although pH should be calculated to the number of significant figures appropriate to the data, the answers are often considerably less reliable than that. One reason for this poor reliability is that in- teractions between the ions in solution are not taken into account. This procedure is illustrated in Examples 6D.1 and 6D.2.