In the human body torques produced by the contraction of muscles cause some bones to rotate at joints. For example, when you lift something with your forearm, a torque is applied to the lower arm by the biceps muscle ( Figure 8.4). With the axis of rotation through the elbow joint and the muscle attached \(4.0 \mathrm{~cm}\) from the joint, what are the magnitudes of the muscle torques for cases (a) and (b) in Figure 8.4 if the muscle exerts a force of \(600 \mathrm{~N}\)?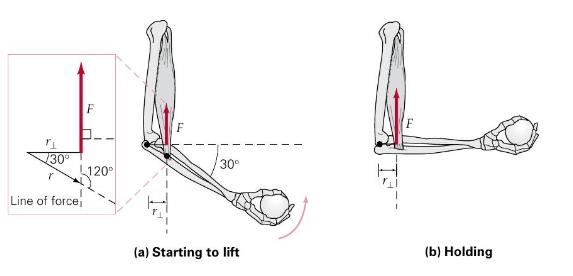

THINKING IT THROUGH. As in many rotational situations, it is important to know the orientations of the \(\overrightarrow{\mathbf{r}}\) and \(\overrightarrow{\mathbf{F}}\) vectors so that the angle between them can be found to determine the lever arm. Note in the inset in Figure 8.4a that if the tails of the \(\overrightarrow{\mathbf{r}}\) and \(\overrightarrow{\mathbf{F}}\) vectors were put together, the angle between them would be greater than \(90^{\circ}\); that is \(30^{\circ}+90^{\circ}=120^{\circ}\). In Figure \(8.4 \mathrm{~b}\), the angle is \(90^{\circ}\). This Example demonstrates an important point, namely that \(\theta\) is the angle between the radial vector \(\overrightarrow{\mathbf{r}}\) and the force vector \(\overrightarrow{\mathbf{F}}\).

Transcribed Image Text:

30 Line of force 30 120 (a) Starting to lift (b) Holding