A central composite design is run in a chemical vapor deposition process, resulting in the experimental data
Question:
A central composite design is run in a chemical vapor deposition process, resulting in the experimental data shown on the next page. Four experimental units were processed simultaneously on each run of the design, and the responses are the mean and variance of thickness, computed across the four units.
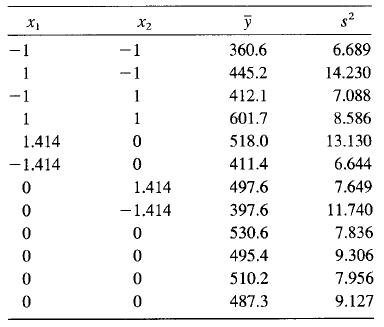
(a) Fit a model to the mean response. Analyze the residuals.
(b) Fit a model to the variance response. Analyze the residuals.
(c) Fit a model to ln(s2). Is this model superior to the one you found in part (b)?
(d) Suppose you want the mean thickness to be in the interval 450 ± 25. Find a set of operating conditions that achieve this objective and simultaneously minimize the variance.
(e) Discuss the variance minimization aspects of part (d). Have you minimized the total process variance?
Step by Step Answer:
Based on the information given youve provided a table of experimental data from a chemical vapor deposition process The table appears to show the coded levels of two factors x1 and x2 along with corre...View the full answer
