For the stress state given in Example 3.1, determine the von Mises and octahedral stresses defined in Section 3.5.

Data from example 3.1

For the following state of stress, determine the principal stresses and directions and find the traction vector on a plane with unit normal n = (0, 1, 1)/ √2:

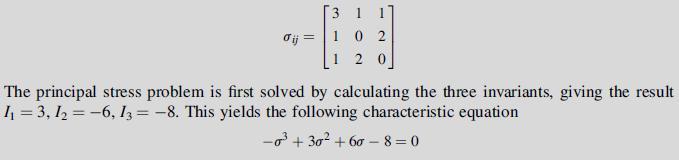
The roots of this equation are found to be σ = 4, 1, –2. Back substituting the first root into the fundamental system [see (1.6.1)] gives:

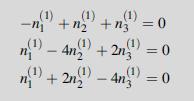
Equation 1.6.1

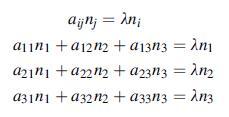
Solving this system, the normalized principal direction is found to be n ⁽¹⁾ = (2, 1, 1)/ √6. In similar fashion the other two principal directions are n ⁽²⁾ = (–1, 1, 1)/ √3, n ⁽³⁾ = (0, –1, 1)/ √2. The traction vector on the specified plane is calculated by using the relation:

Transcribed Image Text:

dij 1 0 2 120 The principal stress problem is first solved by calculating the three invariants, giving the result 1 = 3, 1 = 6, 13 = -8. This yields the following characteristic equation -0 +30 +60-8=0