It was discussed in Section 3.4 that for the case of ranked principal stresses ( 1 >
Question:
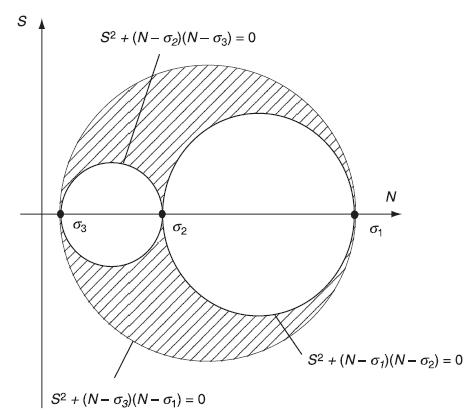
It was discussed in Section 3.4 that for the case of ranked principal stresses (σ1 > σ2 > σ3), the maximum shear stress was given by Smax = (σ1– σ3)/2, which was the radius of the largest Mohr circle shown in Fig. 3.7. For this case, show that the normal stress acting on the plane of maximum shear is given by N = (σ1 + σ3)/2. Finally, using relations (3.4.9) show that the components of the unit normal vector to this plane are ni =± (1, 0, 1)/√2 . This result implies that the maximum shear stress acts on a plane that bisects the angle between the directions of the largest and the smallest principal stress.
Fig 3.7
Equation 3.4.9
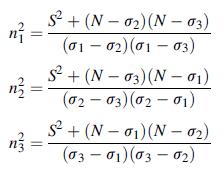
Fantastic news! We've Found the answer you've been seeking!
Step by Step Answer:
Related Book For 

Elasticity Theory Applications And Numerics
ISBN: 9780128159873
4th Edition
Authors: Martin H. Sadd Ph.D.
Question Posted:





