Using polar coordinates and the basic results of Exercise 9.6, formulate the torsion of a cylinder of circular section with radius a, in terms of the usual Prandtl stress function. Note for this case, there will be no warping displacement and ∅ = ∅(r). Show that the stress function is given by:

and the only non-zero stress simplifies to τ_,θz = μαr. Check these results with the solution given for the elliptical section for the case with a = b.

Data from exercise 9.6

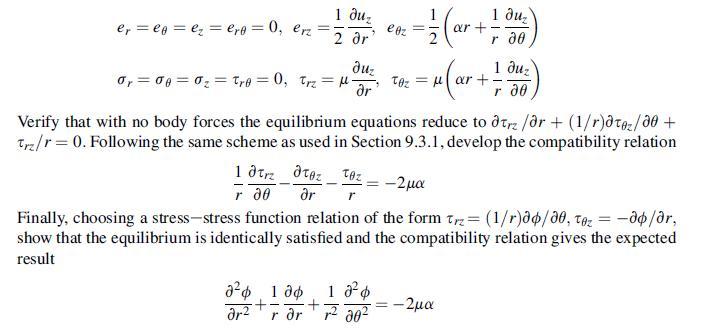
We wish to reformulate the torsion problem using cylindrical coordinates. First show that the general form of the displacements can be expressed as u_r = 0, u_θ = αrz, u_z = u_z(r,θ). Next show that this leads to the following strain and stress fields:

Transcribed Image Text:

2 (203)