Using data for 1901 through 1999, annual earned run averages (ERAs) were tabulated for all Major League
Question:
Using data for 1901 through 1999, annual earned run averages (ERAs) were tabulated for all Major League Baseball (MLB) pitchers for each season in which the player pitched at least 25 innings. For each season, each player’s ERA was converted to a standardized Z value by subtracting the average ERA for all players that year and dividing by the standard deviation of ERAs that year [2]. The annual Z values were grouped according to whether it was the player’s first year in the major leagues, second year, and so on. The average Z value was then calculated for each career year. For example, looking at each player’s first year in the major leagues, the average Z value is −0.176.
a. Use the data in Table 11.3 to estimate the multiple regression equation Z = α +
β1t + β2t2.
b. Are the coefficients of t and t2 statistically significant at the 5 percent level?
c. What do the signs of t and t2 tell you about the shape of the fitted line?
d. What worrisome pattern do you see in the residuals about the fitted line?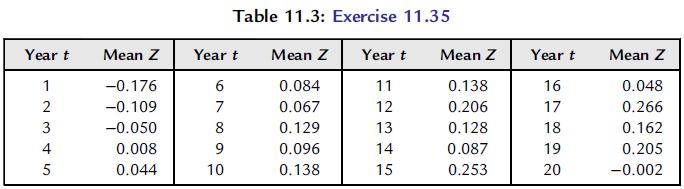
Step by Step Answer:

Essential Statistics Regression And Econometrics
ISBN: 9780123822215
1st Edition
Authors: Gary Smith





