Consider an economy with \(N\) traded securities (among which a risk free asset), \(I\) agents with utility functions of the generalized power form, as in Proposition 4.15, with \(\sum_{i=1}^{I} \gamma_{i}=0\) and identical cautiousness coefficient \(b\), and suppose that all the agents are only endowed with units of traded securities. Consider a Call option written on the \(n\)-th asset, with strike price \(k\), and denote by \(p^{\text {call, } n}\) its price at time \(t=0\). Suppose that

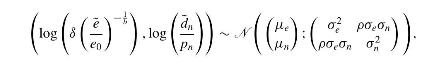
where the random variable \(\tilde{e}\) denotes the random aggregate endowment at \(t=1\) and \(\tilde{d}_{n}\) denotes the random dividend of the \(n\)-th security, for some correlation coefficient \(ho\).
(i) Prove that the equilibrium of the economy can be characterized in terms of the no-trade equilibrium of a single representative agent with a utility function of the generalized power form.

(ii) Show that \[\begin{equation*}
p^{\text {call,n }}=\delta \mathbb{E}\left[\max \left\{\tilde{d}_{n}-k ; 0\right\}\left(\frac{\tilde{e}}{e_{0}}\right)^{-\frac{1}{b}}\right] \tag{4.64}
\end{equation*}\]
(iii) Show that formula (4.64) admits the explicit representation \[\begin{equation*}
p^{\text {call,n }}=p_{n} N\left(\Delta_{1}\right)-\frac{k}{r_{f}} N\left(\Delta_{2}\right) \tag{4.65}
\end{equation*}\]
where \[\begin{aligned}
& \qquad \Delta_{1}:=\frac{\log \left(\frac{p_{n}}{k}\right)+\log \left(r_{f}\right)}{\sigma_{n}}+\frac{\sigma_{n}}{2} \quad \text { and } \Delta_{2}:=\Delta_{1}-\sigma_{n} \\
& \text { and } N(x):=\frac{1}{\sqrt{2 \pi}} \int_{-\infty}^{x} \mathrm{e}^{-z^{2} / 2} d z \end{aligned}\]

Transcribed Image Text:

N ((A) A) (0) (0)) .log