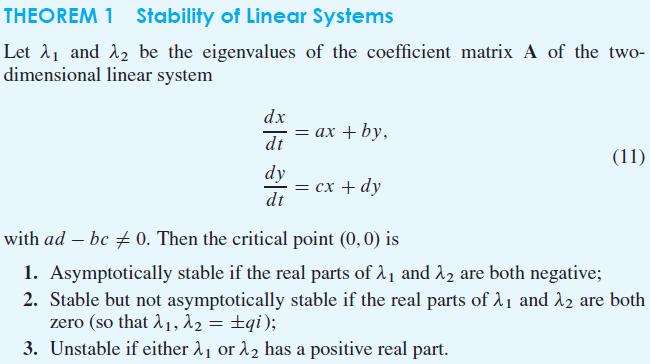
First note that the characteristic equation of the 2 x 2 matrix A can be written in the form λ² - Tλ + D = 0, where D is the determinant of A and the trace T of the matrix A is the sum of its two diagonal elements. Then apply Theorem 1 to show that the type of the critical point (0, 0) of the system x' = Ax is determined—as indicated in Fig. 9.2.15 —by the location of the point (T,D) in the trace-determinant plane with horizontal T -axis and vertical D-axis.

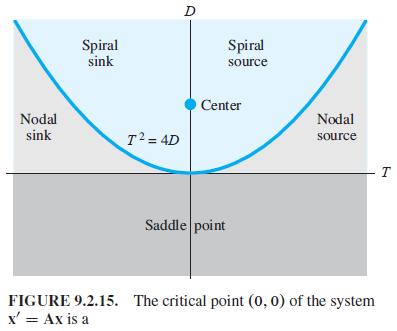
. spiral sink or source if the point (T, D) lies above the parabola T² = 4D but off the D-axis;

. stable center if (T, D) lies on the positive D-axis;

. nodal sink or source if (T, D) lies between the parabola and the T-axis;

. saddle point if (T, D) lies beneath the T-axis.

Transcribed Image Text:

Nodal sink Spiral sink T² = 4D D Spiral source Center Saddle point Nodal source FIGURE 9.2.15. The critical point (0, 0) of the system x' = Ax is a T