Problems 31-38 illustrate ways in which the algebra of matrices is not analogous to the algebra of real numbers.

(a) Suppose that A and B are the matrices of Example 5. Show that (A + B)(A - B) ≠ A² - B².

(b) Suppose that A and B are square matrices with the property that AB = BA. Show that (A + B)(A - B) = A² - B².

Example 5

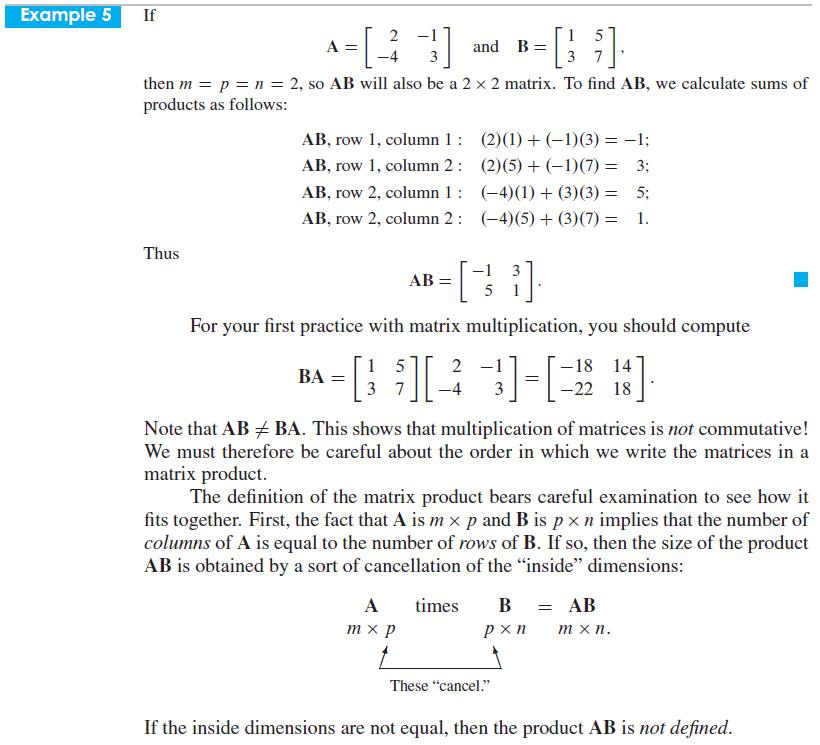
Transcribed Image Text:

Example 5 If 1 A = [ ² ] and B = [] 2 -1 3 37 then m = p = n = 2, so AB will also be a 2 x 2 matrix. To find AB, we calculate sums of products as follows: Thus AB, row 1, column 1: AB, row 1, column 2: AB, row 2, column 1: AB, row 2, column 2: -1 B = [3³] 5 For your first practice with matrix multiplication, you should compute 15 2 [][] 37 -4 BA = (2)(1) + (-1)(3) = -1; (2) (5) + (-1)(7) = 3; (-4) (1) + (3)(3) = 5; (-4) (5) + (3)(7) = 1. AB A mx p 3 = -18 14 {}] -22 18 Note that AB #BA. This shows that multiplication of matrices is not commutative! We must therefore be careful about the order in which we write the matrices in a matrix product. The definition of the matrix product bears careful examination to see how it fits together. First, the fact that A is m x p and B is pxn implies that the number of columns of A is equal to the number of rows of B. If so, then the size of the product AB is obtained by a sort of cancellation of the "inside" dimensions: times B = AB pxn mxn. These "cancel." If the inside dimensions are not equal, then the product AB is not defined.