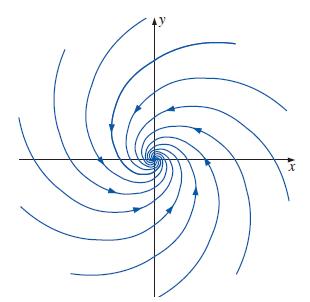
Consider a spiraling line vortex/sink flow in the xy-or r-plane as sketched in Fig. P917. The two-dimensional
Question:
Consider a spiraling line vortex/sink flow in the xy-or rθ-plane as sketched in Fig. P9–17. The two-dimensional cylindrical velocity components (ur, uθ) for this flow field are ur = C/2πr and uθ = Γ/2pr, where C and G are constants (m is negative and Γ is positive). Transform these two-dimensional cylindrical velocity components into two dimensional Cartesian velocity components (u, v). Your final answer should contain no r or θ—only x and y. As a check of your algebra, calculate V2 using Cartesian coordinates, and compare to V2 obtained from the given velocity components in cylindrical components.
Figure 9-17
Fantastic news! We've Found the answer you've been seeking!
Step by Step Answer:
To transform twodimensional cylindrical velocity components ur u into twodimensional Cartesian veloc...View the full answer
Answered By
User l_998468
I have extensive tutoring experience, having worked as a private tutor for over three years. I have tutored students from different academic levels, including high school, undergraduate, and graduate levels. My tutoring experience has taught me to be patient, attentive to student needs, and effective in communicating difficult concepts in simple terms.
I have a strong background in statistics, probability theory, data analysis, and data visualization. I am proficient in using statistical software such as R, Python, and SPSS, which are commonly used in academic research and data analysis. Additionally, I have excellent communication and interpersonal skills, which enable me to establish rapport with students, understand their learning styles, and adapt my teaching approach to meet their needs.
I am passionate about teaching and helping students achieve their academic goals.
0 Reviews
10+ Question Solved
Related Book For 
Fluid Mechanics Fundamentals And Applications
ISBN: 9780073380322
3rd Edition
Authors: Yunus Cengel, John Cimbala
Question Posted:
