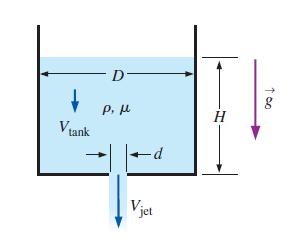
Consider flow of water through a small hole in the bottom of a large cylindrical tank (Fig. P10–13). The flow is laminar everywhere. Jet diameter d is much smaller than tank diameter D, but D is of the same order of magnitude as tank height H. Carrie reasons that she can use the fluid statics approximation everywhere in the tank except near the hole, but wants to validate this approximation mathematically. She lets the characteristic velocity scale in the tank be V = V_tank. The characteristic length scale is tank height H, the characteristic time is the time required to drain the tank t_drain, and the reference pressure difference is ρgH (pressure difference from the water surface to the bottom of the tank, assuming fluid statics). Substitute all these scales into the nondimensionalized incompressible Navier–Stokes equation (Eq. 10–6) and verify by order-of-magnitude analysis that for d ≪ D, only the pressure and gravity terms remain. In particular, compare the order of magnitude of each term and each of the four non-dimensional parameters St, Eu, Fr, and Re.

Under what criteria is Carrie’s approximation appropriate?

Eq. 10–6

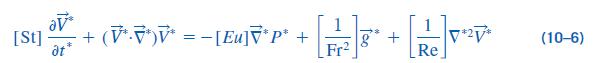
FIGURE P10–13

Transcribed Image Text:

Vin jet 2 VgH.