In Chap. 9 (Example 915), we generated an exact solution of the NavierStokes equation for fully developed
Question:
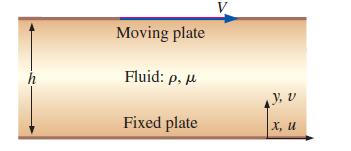
In Chap. 9 (Example 9–15), we generated an “exact” solution of the Navier–Stokes equation for fully developed Couette flow between two horizontal flat plates (Fig. P10–12), with gravity acting in the negative z-direction (into the page of Fig. P10–12). We used the actual pressure in that example. Repeat the solution for the x-component of velocity u and pressure P, but use the modified pressure in your equations. The pressure is P0 at z = 0. Show that you get the same result as previously. Discuss.
FIGURE P10–12
Data from Example 9-15
Consider steady, incompressible, laminar flow of a Newtonian fluid in the narrow gap between two infinite parallel plates (Fig. 9–57). The top plate is moving at speed V, and the bottom plate is stationary. The distance between these two plates is h, and gravity acts in the negative z-direction (into the page in Fig. 9–57). There is no applied pressure other than hydrostatic pressure due to gravity. This flow is called Couette flow. Calculate the velocity and pressure fields, and estimate the shear force per unit area acting on the bottom plate.
FIGURE 9–57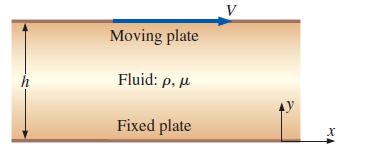
Step by Step Answer:

Fluid Mechanics Fundamentals And Applications
ISBN: 9780073380322
3rd Edition
Authors: Yunus Cengel, John Cimbala





