The pressure drop P = P 1 P 2 through a long section of round pipe

The pressure drop ΔP = P₁ – P₂ through a long section of round pipe can be written in terms of the shear stress τ_w along the wall. Shown in Fig. P7–97 is the shear stress acting by the wall on the fluid. The shaded region is a control volume composed of the fluid in the pipe between axial locations 1 and 2. There are two dimensionless parameters related to the pressure drop: the Euler number Eu and the Darcy friction factor f. 

(a) Using the control volume sketched in Fig. P7–97, generate a relationship for f in terms of Eu (and any other properties or parameters in the problem as needed). 

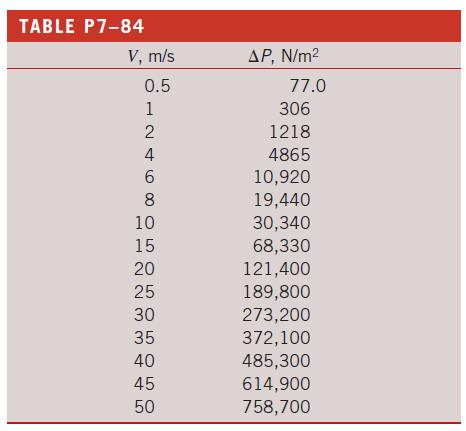
(b) Using the experimental data and conditions of Prob. 7–84 (Table P7–84), plot the Darcy friction factor as a function of Re. Does f show Reynolds number independence at large values of Re? If so, what is the value of f at very high Re?

FIGURE P7–97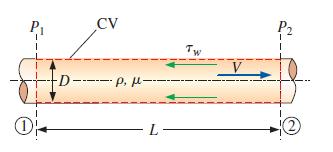

Data from Problem 84

Water at 20°C flows through a long, straight pipe. The pressure drop is measured along a section of the pipe of length L = 1.3 m as a function of average velocity V through the pipe (Table P7–84). The inner diameter of the pipe is D = 10.4 cm. 

Nondimensionalize the data and plot the Euler number as a function of the Reynolds number. Has the experiment been run at high enough speeds to achieve Reynolds number independence? 

Extrapolate the experimental data to predict the pressure drop at an average speed of 80 m/s.

Transcribed Image Text:

О CV -D ----- P, M----- L- (2)