Optical fibers are made by drawing the hot glass from a furnace as shown in Figure P15.7.
Question:
Optical fibers are made by drawing the hot glass from a furnace as shown in Figure P15.7. Heat, generated in the furnace, is transferred by conduction along the drawn glass fiber, by convection to the atmosphere at an ambient temperature, \(T_{\infty}\), through a heat-transfer coefficient, \(\bar{h}\), and by radiation to the ambient air. The fiber is pulled at a constant velocity, \(\overline{v_{x}}\). The fiber temperature is \(T(x)\), and the cross-sectional area and perimeter are \(A_{c}\), and \(P_{d}\) respectively. Assuming the fiber may be treated as a fin (1-D conduction) under steady-state conditions, start with an energy balance on a control volume (fixed in space) of length \(\Delta x\) and show that the following equation for \(T(x)\) results. (Conductivity, \(k\), density, \(ho\), and heat capacity, \(C_{p}\), are known. The air and fiber can be assumed to be blackbodies.)
\[\frac{d^{2} T}{d x^{2}}-\frac{ho C_{p} \overline{v_{x}}}{k} \frac{d T}{d x}-\frac{\bar{h} P_{d}}{k A_{c}}\left(T-T_{\infty}\right)-\frac{\sigma P_{d}}{k A_{c}}\left(T^{4}-T_{\infty}^{4}\right)=0\]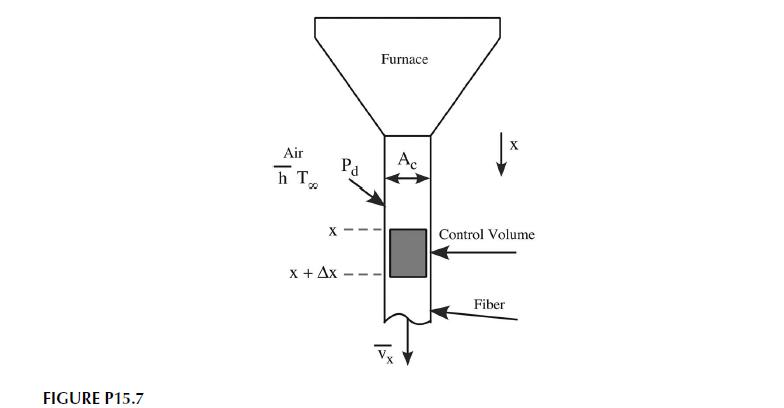
Step by Step Answer:






