(a) From Maxwell equations, where E and H denote the electric and magnetic fields in a plasma,...
Question:
(a) From Maxwell equations,
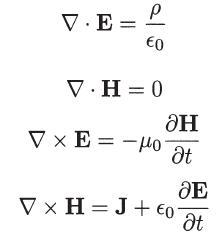
where E and H denote the electric and magnetic fields in a plasma, p denotes the electric charge density nq, and J the electric current density nqu, show that
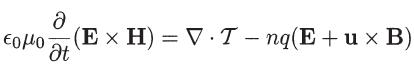
where ∈0μ0 (E x H) is the electromagnetic momentum density, and T is the electromagnetic stress dyad whose components are given by
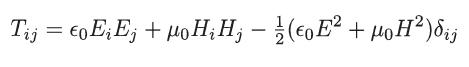
where δij is the Kronecker delta.
(b) From this equation, which expresses conservation of the electromagnetic momentum density, and the continuity equation
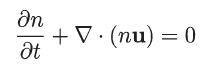
show that the momentum transport equation
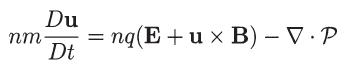
can be written in the form
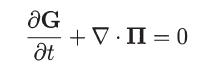
where G denotes the total momentum density
![]()
and Π is the total momentum flux dyad (rate of transport of momentum through unit area)
![]()
(c) Using Maxwell curl equations, show that the energy transport equation
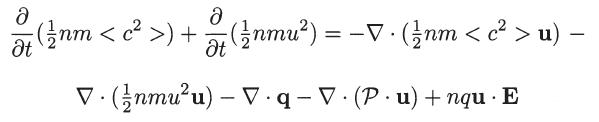
can be written in the form [note that u · (u x B) = 0]
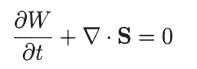
where W denotes the total energy density
![]()
and S is the total energy flux (power per unit area)
![]()
Step by Step Answer:






