Consider an electron gas immersed in a uniform magnetostatic field B 0 and characterized by the following
Question:
Consider an electron gas immersed in a uniform magnetostatic field B0 and characterized by the following modified Maxwellian distribution function:
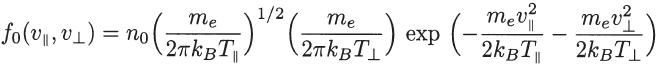
Use this distribution function in the dispersion relation for the right circularly polarized transverse wave propagating along B0, given in (2.69), and evaluate the integrals to obtain the following dispersion relation

where
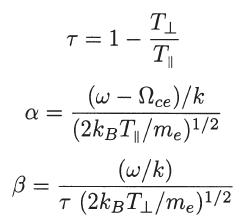
Analyze this dispersion relation to verify the existence or not of instabilities (positive imaginary part of ω) and/or damping (negative imaginary part of ω) of the wave amplitude, considering the propagation coefficient k = kẑ to be real. Determine the cyclotron damping coefficient. Analyze also the results considering the isotropic case for which T∥ = T⊥.
Equation 2.69.
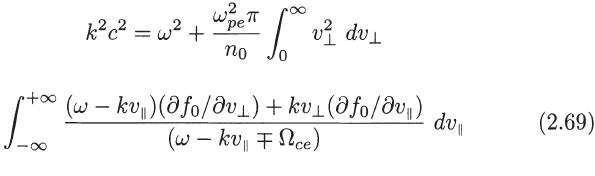
Step by Step Answer:






