Walker and Beebe (2002) demonstrated a way to pump liquid through small tubes without the need for moving parts, and their idea has been applied to pointof- care diagnostics and other microfluidic devices. The concept is illustrated in Fig. P8.16. Liquid drops of radius R0 and RL are in contact with the ends of a horizontal capillary tube of radius R and length L. The flow is created by the unequal drop radii.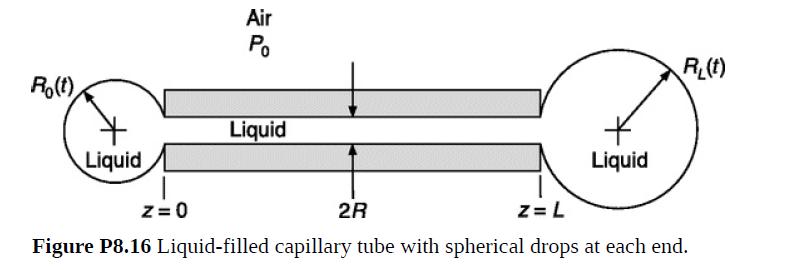

(a) If R_L > R₀, as shown, in which direction will the liquid flow?

(b) Assuming that the flow in the tube is fully developed and pseudosteady, determine the instantaneous flow rate Q(t).

(c) If R₀ ≫ R and R_L ≫ R, such that both drops are nearly complete spheres, derive the differential equations that govern R₀(t) and R_L(t). (You are not asked to solve them.)

(d) Sketch the final appearance of the two drops. Would the equations in part (c) be sufficient to determine the time t_p at which the flow will stop?

Transcribed Image Text:

Ro(t) Liquid Z=0 Air Po Liquid Liquid R₂(t) 2R Z=L Figure P8.16 Liquid-filled capillary tube with spherical drops at each end.