(a) Show that the set of all square-integrable functions is a vector space (refer to Section A.1...
Question:
(a) Show that the set of all square-integrable functions is a vector space (refer to Section A.1 for the definition). The main point is to show that the sum of two square-integrable functions is itself square-integrable. Use Equation 3.7. Is the set of all normalized functions a vector space?
(b) Show that the integral in Equation 3.6 satisfies the conditions for an inner product (Section A.2).
Equation 3.6

Equation 3.7
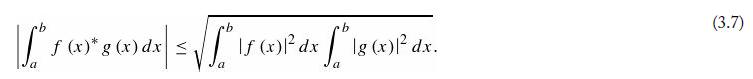
Fantastic news! We've Found the answer you've been seeking!
Step by Step Answer:
Related Book For 

Introduction To Quantum Mechanics
ISBN: 9781107189638
3rd Edition
Authors: David J. Griffiths, Darrell F. Schroeter
Question Posted:





