Estimate the correction to the ground state energy of hydrogen due to the finite size of the nucleus. Treat the proton as a uniformly charged spherical shell of radius b, so the potential energy of an electron inside the shell is constant: -e²/(4π∈₀b); this isn’t very realistic, but it is the simplest model, and it will give us the right order of magnitude. Expand your result in powers of the small parameter (b/a), where a is the Bohr radius, and keep only the leading term, so your final answer takes the form

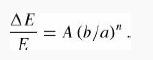
Your business is to determine the constant A and the power n. Finally, put in b ≈ 10^-15 m (roughly the radius of the proton) and work out the actual number. How does it compare with fine structure and hyperfine structure?

Transcribed Image Text:

ΔΕ = Ah/a)" . E