Consider a functional of the form [T(F)=int_{-infty}^{infty} int_{-infty}^{infty} hleft(x_{1}, x_{2}ight) d Fleft(x_{1}ight) d Fleft(X_{2}ight)] where (F in
Question:
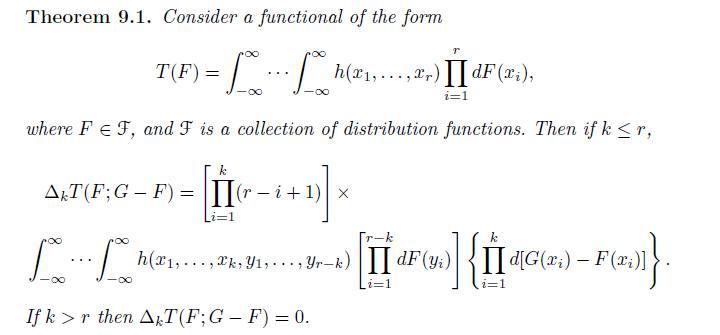
Consider a functional of the form
\[T(F)=\int_{-\infty}^{\infty} \int_{-\infty}^{\infty} h\left(x_{1}, x_{2}ight) d F\left(x_{1}ight) d F\left(X_{2}ight)\]
where \(F \in \mathcal{F}\), a collection of distribution functions. Find an expression for the \(k^{\text {th }}\) Gâteaux differential of \(T(F)\) without using the assumption that \(h\) is a symmetric function of its arguments. Compare this result to that of Theorem 9.1.
Fantastic news! We've Found the answer you've been seeking!
Step by Step Answer:
Related Book For 

Question Posted:





