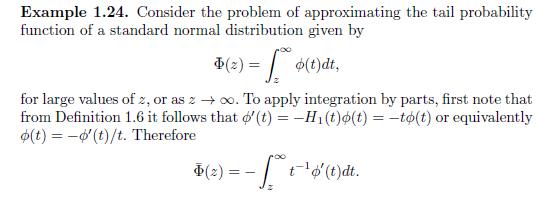
Consider the approximation for the normal tail integral \(\bar{\Phi}(z)\) studied in Example 1.24 given by

\[\bar{\Phi}(z) \simeq z^{-1} \phi(z)\left(1-z^{-2}+3 z^{-4}-15 z^{-6}+105 z^{-8}ight) .\]

A slight rearrangement of the approximation implies that

\[\frac{z \bar{\Phi}(z)}{\phi(z)} \simeq 1-z^{-2}+3 z^{-4}-15 z^{-6}+105 z^{-8}\]

Define \(S_{1}(z)=1-z^{-2}, S_{2}(z)=1-z^{-2}+3 z^{-4}, S_{3}(z)=1-z^{-2}+3 z^{-4}-\) \(15 z^{-6}\) and \(S_{4}(z)=1-z^{-2}+3 z^{-4}-15 z^{-6}+105 z^{-8}\), which are the successive approximations of \(z \bar{\Phi}(z) / \phi(z)\). Using R, compute \(z \bar{\Phi}(z) / \phi(z), S_{1}(z)\), \(S_{2}(z), S_{3}(z)\), and \(S_{4}(z)\) for \(z=1, \ldots, 10\). Comment on which approximation performs best for each value of \(z\) and whether the approximations become better as \(z\) becomes larger.

Transcribed Image Text:

Example 1.24. Consider the problem of approximating the tail probability function of a standard normal distribution given by (z) = (t)dt, for large values of z, or as zoo. To apply integration by parts, first note that from Definition 1.6 it follows that o' (t) = -H(t)o(t) = -to(t) or equivalently (t)='(t)/t. Therefore 4(2) =- to' (t)dt.