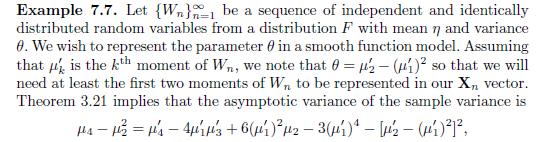
Let \(\left\{W_{n}ight\}_{n=1}^{\infty}\) be a sequence of independent and identically distributed random variables from a distribution \(F\) with mean \(\eta\) and variance \(\theta\). Prove that the parameter \(\theta\) can be represented in the smooth function model with \(d=4, \mathbf{X}_{n}^{\prime}=\left(W_{n}, W_{n}^{2}, W_{n}^{3}, W_{n}^{4}ight)\) for all \(n \in \mathbb{N}, g(\mathbf{x})=x_{2}-\left(x_{1}ight)^{2}\), and

\[h(\mathbf{x})=x_{4}-4 x_{1} x_{3}+6 x_{1}^{2} x_{2}-3 x_{1}^{4}-\left(x_{2}-x_{1}^{2}ight)^{2},\]

where \(\mathbf{x}^{\prime}=\left(x_{1}, x_{2}, x_{3}, x_{4}ight)\). This will verify the results of Example 7.7.

Transcribed Image Text:

Example 7.7. Let {Wn} be a sequence of independent and identically distributed random variables from a distribution F with mean 7 and variance 8. We wish to represent the parameter in a smooth function model. Assuming that is the kth moment of Wn, we note that =2-(1) so that we will need at least the first two moments of W, to be represented in our X, vector. Theorem 3.21 implies that the asymptotic variance of the sample variance is -=-43 +6(1)2-3(1) - [2-(1)],