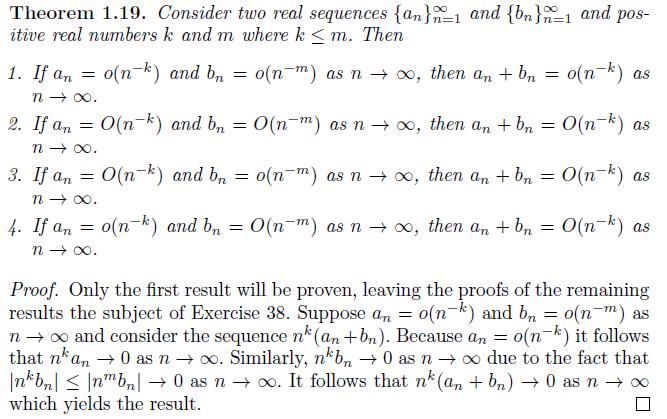
Prove the remaining three results of Theorem 1.19. That is, consider two real sequences \(\left\{a_{n}ight\}_{n=1}^{\infty}\) and \(\left\{b_{n}ight\}_{n=1}^{\infty}\) and positive integers \(k\) and \(m\) where \(k \leq m\). Then

a. Suppose \(a_{n}=O\left(n^{-k}ight)\) and \(b_{n}=O\left(n^{-m}ight)\) as \(n ightarrow \infty\). Then prove that \(a_{n}+b_{n}=O\left(n^{-k}ight)\) as \(n ightarrow \infty\).

b. Suppose \(a_{n}=O\left(n^{-k}ight)\) and \(b_{n}=o\left(n^{-m}ight)\) as \(n ightarrow \infty\). Then prove that \(a_{n}+b_{n}=O\left(n^{-k}ight)\) as \(n ightarrow \infty\).

c. Suppose \(a_{n}=o\left(n^{-k}ight)\) and \(b_{n}=O\left(n^{-m}ight)\) as \(n ightarrow \infty\). Then prove that \(a_{n}+b_{n}=O\left(n^{-k}ight)\) as \(n ightarrow \infty\).

Transcribed Image Text:

Theorem 1.19. Consider two real sequences {an} and {bn}1 and pos- itive real numbers k and m where k