Consider a supply and demand model written in its most general implicit form, using capital Greek letters for the unknown parameters and (E i ) for the random errors, a Multiply each equation by 3 Do they remain true b Multiply the demand equation by ( 1 Gamma 11 ) Does it remain true c Define ( al...

The Answer is in the image, click to view ...

Consider a supply and demand model written in its most general implicit form, using capital Greek letters

Question:

Consider a supply and demand model written in its most general implicit form, using capital Greek letters for the unknown parameters and \(E_{i}\) for the random errors,

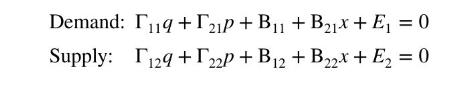
image text in transcribed

a. Multiply each equation by 3 . Do they remain true?

b. Multiply the demand equation by \(-1 / \Gamma_{11}\). Does it remain true?

c. Define \(\alpha_{21}=-\Gamma_{21} / \Gamma_{11}, \beta_{11}=-\mathrm{B}_{11} / \Gamma_{11}, \beta_{21}=-\mathrm{B}_{21} / \Gamma_{11}, e_{1}=-E_{1} / \Gamma_{11}\) and write the demand equation with \(q\) on the left-hand side and the remaining terms on the right-hand side. By choosing \(q\) to be on the left-hand side of the equation, we have chosen a normalization rule.

d. Repeat the process for the supply equation, beginning by multiplying through by \(-1 / \Gamma_{22}\), and obtain the normalized supply curve with

Write the normalized supply equation with \(p\) on the left-hand side and the remaining terms on the right side.

e. Mathematically, in a system of jointly determined variables, it does not matter which variable appears on the left side of each normalized equation. True or false?

Fantastic news! We've Found the answer you've been seeking!