Using the data in cps 5 obtain OLS estimates of the wage equation

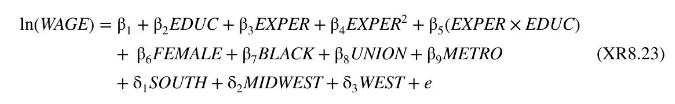
a. Interpret the coefficient of UNION. Test the null hypothesis that the coefficient of UNION is less than or equal to zero, against the alternative that is positive. What do you conclude?

b. Test for the presence of heteroskedasticity related to the variables UNION and METRO using the \(N R^{2}\) test. What do you conclude at the \(1 \%\) level of significance?

c. Regress the squared least squares residuals, \(\hat{e}_{i}^{2}\), from (a) on EDUC, UNION, and METRO. Also regress \(\ln \left(\hat{e}_{i}^{2}\right)\) on EDUC, UNION, and METRO. What do these results suggest about the effect of UNION membership on the variation in the random error? What do these results suggest about the effect of METRO on the variation in the random error?

d. Hypothesize that \(\sigma_{i}^{2}=\sigma^{2} \exp \left(\alpha_{2} E D U C+\alpha_{3} U N I O N+\alpha_{4} M E T R O\right)\). Find generalized least squares estimates of the wage equation. For the coefficient of UNION, compare the estimates and standard errors with those obtained from OLS estimation of (XR8.23) with heteroskedasticity robust standard errors.

Transcribed Image Text:

In(WAGE) = B + EDUC + BEXPER + BEXPER + s(EXPER EDUC) +B6FEMALE + B,BLACK+ 8 UNION + ,METRO +8,SOUTH+8,MIDWEST+8, WEST + e (XR8.23)