According to Theorem 12, a linearly independent set {v₁,...,v_k in Rⁿ can be expanded to a basis for Rⁿ. One way to do this is to create A = [v₁ ... v_k e₁ ... e_n], with e₁,...,e_n  the columns of the identity matrix; the pivot columns of A form a basis for Rⁿ. 

a. Use the method described to extend the following vectors to a basis for R⁵:

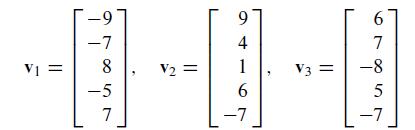
b. Explain why the method works in general: Why are the original vectors V,..., Vk included in the basis found for Col A? Why is Col A = Rⁿ?

Data from in Theorem 12

Transcribed Image Text:

VI V1 = -9 -7 8 -5 7 V2 || 9 4 1 6 -7 V3 || 6 7 -8 5 -7]