Implement via Monte Carlo a long-dated FX model with stochastic interest rates and stochastic vol. The parameterisation

Question:

Implement via Monte Carlo a long-dated FX model with stochastic interest rates and stochastic vol. The parameterisation in Question 2 of Chapter 10 is reasonable for interest rates and spot FX processes. Use a Heston-style stochastic vol. Take vol of variance of 100%, mean reversion speed of 100%, correlation of 0% as benchmark. Convince yourself by varying the stochastic vol parameters that the impact on smile for longexpiry options (i.e. over 20 years) is moderated by the stochastic interest rates.

Question 2

Consider the same parameterisation as in Question 1 but to be more specific, say the HJM processes correspond to a Hull-White model with constant vol of 0.7% and mean reversion speed of 2%. Take lognormal spot FX vol as 15%. The correlation for the domestic-foreign rates is 30% and other correlations are 0%. Using the formula for forward vol in Section 10.1.1, determine how much of forward variance comes from the interest rates component and how much from the spot component for forward starting times 1y, 5y, 10y and expiries 1y, 5y,10y, 20y, 30y.

Question 1

Consider the following parameterisation based on Gaussian interest rates and lognormal spot FX : dS_t = (rt – r^ƒ_t) S_tdt + 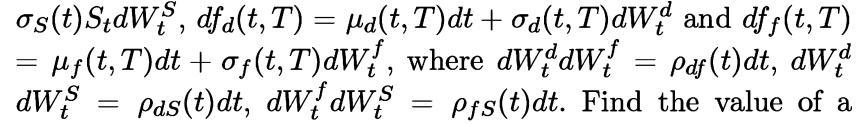os(t) StdWS, dfa(t,T) = a(t, T)dt + od(t, T)dW? and dff(t,T) = f(t, T)dt + of(t, T)dW!, where dwddw! = quanto Libor rate, i.e. where the Libor rate L_T (set at time T, with accrual fraction Ƭ and paid at time T + Ƭ) is in the foreign currency but paid in the domestic currency without conversion at the FX rate.