Take the actual SABR formula per Section 6.2.1 with 0 = 0.5%, = 0,

Take the actual SABR formula per Section 6.2.1 with σ₀ = 0.5%, β = 0, ρ = −25%, ν = 30%, and F = 4%. By considering an expiry of 20 years and strikes at 10 basis points increments from 1 % to 2 % inclusive, try and construct an arbitrage involving butterflies (i.e. long a call at strike K − δ short two calls at strike K and long a call at strike K + δ where δ > 0). This is an illustration of the problem of negative densities under the SABR model for very low strikes and long enough expiry.

Section 6.2.1

Transcribed Image Text:

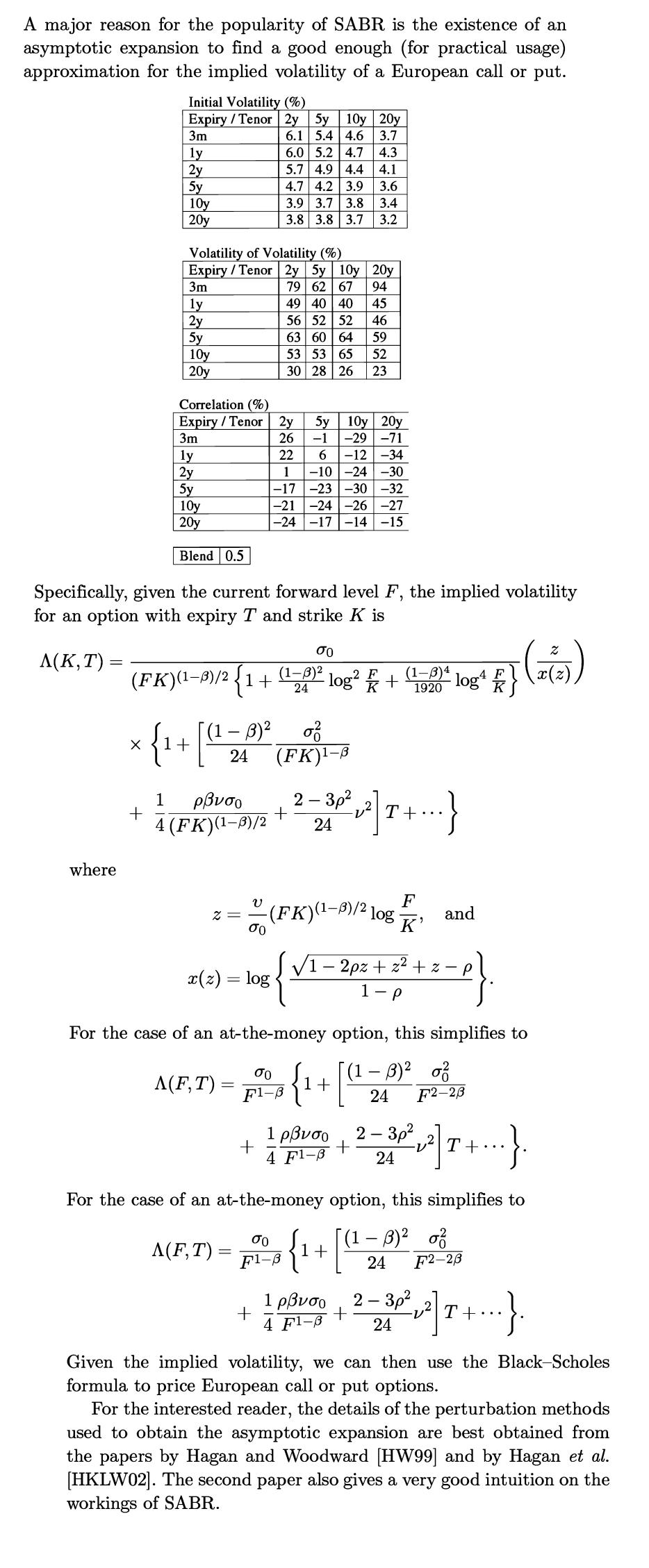
A major reason for the popularity of SABR is the existence of an asymptotic expansion to find a good enough (for practical usage) approximation for the implied volatility of a European call or put. where Initial Volatility (%) Expiry / Tenor 2y 5y 10y 20y 3m 6.1 5.4 4.6 3.7 6.0 5.2 4.7 4.3 5.7 4.9 4.4 4.1 4.7 4.2 3.9 3.6 3.9 3.7 3.8 3.4 3.8 3.8 3.7 3.2 X ly 2y 5y + 10y 20y Volatility of Volatility (%) Expiry / Tenor 2y 5y 10y 3m ly 2y 5y 10y 20y ly 2y 5y 10y 20y Blend 0.5 (FK)(1-3)/2 1 + Correlation (%) Expiry / Tenor 2y 3m 26 1+ (1 - 3)² Specifically, given the current forward level F, the implied volatility for an option with expiry T and strike K is A(K,T) = [(₁ 24 1 ρβυσο 4 (FK)(1-3)/2 z = V σο A(F,T) = 20y 79 62 67 94 49 40 40 x(z) = log 56 52 52 63 60 64 53 53 65 52 30 28 26 23 5y 10y 20y -1 -29 -71 22 6 -12 -34 -10-24 -30 -17 -23 -30 -32 1 -21 -24-26 -27 -24-17-14 -15 σο (1-3)² 2 F (1-3)4 log² + 24 1920 0² (FK)¹-B σο F1-3 + 45 46 59 2-3p² 24 F (FK)(¹-0)/2 log ¹ and √1-2pz +z²+z-p 1+ 2², ²] T +...} For the case of an at-the-money option, this simplifies to σο A(F,T) = ¡{¹+ F1-B 1-p 1ρβνσο + + 4 F1-B 4 F log ¹ K} (x(3)) - 24 F2-28 + + ²] +}. 1ρβνσο + 4 F1-8 2-3p² 24 For the case of an at-the-money option, this simplifies to (1 - 3)² o 24 F2-23 2 - 30/²2, 2] T +... }. 24 Given the implied volatility, we can then use the Black-Scholes formula to price European call or put options. For the interested reader, the details of the perturbation methods used to obtain the asymptotic expansion are best obtained from the papers by Hagan and Woodward [HW99] and by Hagan et al. [HKLW02]. The second paper also gives a very good intuition on the workings of SABR.