Let the exchange rate process X(t), T -maturity domestic and foreign bond processes B d (t, T

Question:

Let the exchange rate process X(t), T -maturity domestic and foreign bond processes B_d (t, T ) and B_f (t, T ) be the same as those defined in Problem 8.9. Find the time-t value of the LIBOR spread option which pays at time T + δ

a [La (T; 8) - Lf (T; 8)],

where t f (T ; δ) are the domestic and foreign LIBORs over the time interval (T, T + δ], respectively, observed at time T.

Problem 8.9.

Let X(t) denote the exchange rate process in units of the domestic currency per one unit of the foreign currency, and let r_d (t) and r_f (t) denote the domestic and foreign riskless interest rate, respectively. Also, let B_d (t, T) and B_f (t, T) denote the T-maturity domestic and foreign bond price processes, respectively, t ≤ T . Under the domestic risk neutral measure Q_d , the dynamics of X(t) and B_d (t, T′) are governed by

dX(t) n = X(1) {[tra(1) r(0)] dr + o{(1) dz{{1}} i=1 Barrawa+Eranazion] 1(t) dt + o(t, T) dZi (t) d Ba(t,

where (Z¹_d (t)···Zⁿ_d (t))^T is an n-dimensional Brownian process under Q_d. Let (σ¹_f (t, T )··· σⁿ_f (t, T ))^T be the vector volatility function of B_f (t, T). Show that the dynamics of B_f (t, T) under Q_d is given by

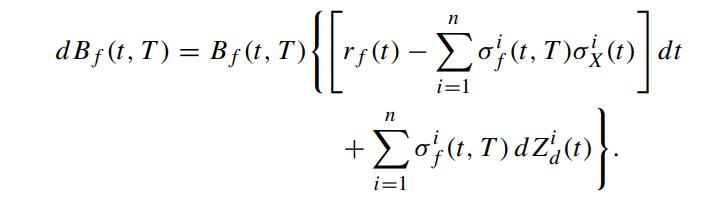dBf(t,T) = Bf(t,T){ f(t) - ,() dt i=1 + n i=1 By using the T-maturity domestic bond price B_d (t, T) as the numeraire, find the Radon–Nikodym derivative that effects the change of measure from Q_d to the T -maturity domestic forward measure Q^T_d . Solve for the solution of the exchange rate process (Nielsen and Sandmann, 2002).