Use the Fourier method to deduce the von Neumann stability condition for (i) the JarrowRudd binomial scheme
Question:
Use the Fourier method to deduce the von Neumann stability condition for (i) the Jarrow–Rudd binomial scheme (see Problem 6.1), (ii) the Kamrad– Ritchken trinomial scheme, and (iii) the explicit FTCS scheme [see (6.2.2)].
Problem 6.1
Instead of the tree-symmetry condition: u = 1/d [see (6.1.1c)], Jarrow and Rudd (1983) chose the third condition to be p = 1/2. By solving together with (6.1.1a,b), show that
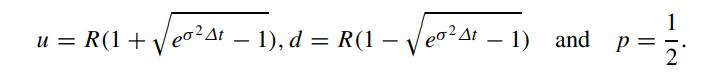
Fantastic news! We've Found the answer you've been seeking!
Step by Step Answer:
Related Book For 

Question Posted:





