Let ((Omega, mathscr{A}, mathbb{P})) be a probability space and let (left(xi_{n}ight)_{n in mathbb{N}}) be a sequence of
Question:
Let \((\Omega, \mathscr{A}, \mathbb{P})\) be a probability space and let \(\left(\xi_{n}ight)_{n \in \mathbb{N}}\) be a sequence of independent random variables with \(\xi_{n} \in \mathcal{L}^{2}(\mathscr{A}), \int \xi_{n} d \mathbb{P}=0\) and \(\int \xi_{n}^{2} d \mathbb{P}=\sigma_{n}^{2}\). Then set \(\mathscr{A}_{n}:=\sigma\left(\xi_{1}, \xi_{2}, \ldots, \xi_{n}ight)\) and \(A_{n}:=\sigma_{1}^{2}+\cdots+\sigma_{n}^{2}\). Show that
\[M_{n}:=S_{n}^{2}-A_{n}=\left(\sum_{i=1}^{n} \xi_{i}ight)^{2}-\sum_{i=1}^{n} \sigma_{i}^{2}\]
is a martingale.
[ use formulae (23.6) and (23.7) and Remark 23.2 (ii).]
Equation 23.6
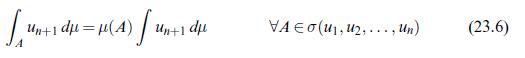
Equation 23.7
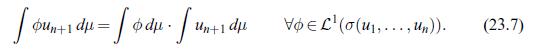
Remark 23.2
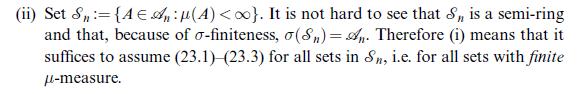
Fantastic news! We've Found the answer you've been seeking!
Step by Step Answer:
Related Book For 

Question Posted:





