Let u , w 0 u , w 0 be measurable functions on a
Question:
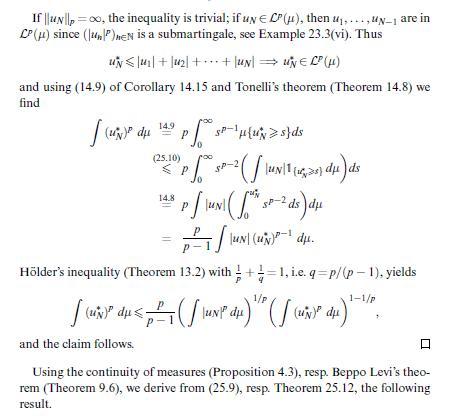
Let u,w⩾0 be measurable functions on a σ-finite measure space (X,A,μ).
(i) Show that tμ{u⩾t}⩽∫{u⩾t}wdμ for all t>0 implies that
∫updμ⩽pp−1∫up−1wdμ∀p>1
(ii) Assume that u,w∈Lp. Conclude from (i) that ∥u∥p⩽(p/(p−1))∥w∥p for p>1.
[use the technique of the proof of Theorem 25.12 ; for (ii) use Hölder's inequality.]
Data from theorem 25.12
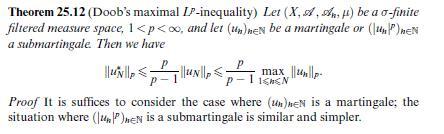
Fantastic news! We've Found the answer you've been seeking!
Step by Step Answer:
Related Book For 

Question Posted:





