Let (u:[a, b] ightarrow mathbb{R}) be a continuous function. Show that (x mapsto int_{[a, x]} u(t) d
Question:
Let \(u:[a, b] ightarrow \mathbb{R}\) be a continuous function. Show that \(x \mapsto \int_{[a, x]} u(t) d t\) is everywhere differentiable and find its derivative. What happens if we assume only that \(u \in \mathcal{L}^{1}(d t)\) ? [ consider Theorem 25.20.]
Data from theorem 25.20
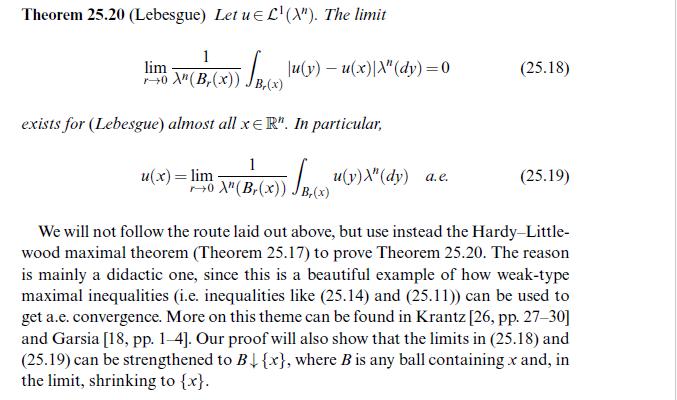
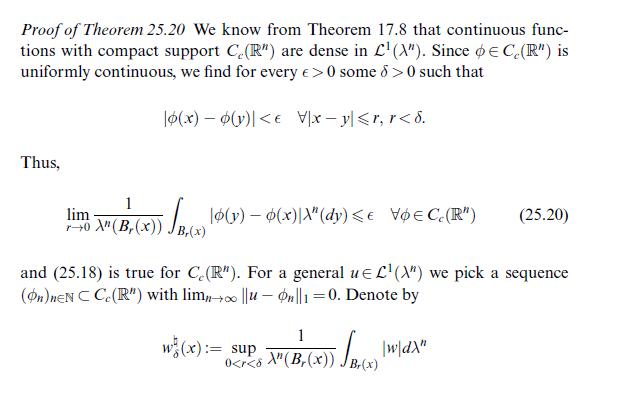
![the restricted maximal function. Since (u-on) 3] 3} } + X"{x:on(x) - u(x) > } Cn "||un||1+0+||on-u||1, where](https://dsd5zvtm8ll6.cloudfront.net/images/question_images/1705/9/2/5/93965ae5d33345871705925938205.jpg)
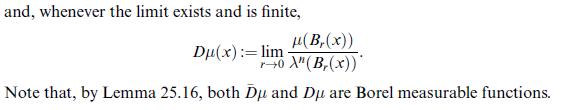
Transcribed Image Text:
Theorem 25.20 (Lebesgue) Let u L (X"). The limit 1 lim X" (B,(x)) , \u(v) u(x)[X" (dy) =0 B.(x) exists for (Lebesgue) almost all xER". In particular, 1 = lim X" (B(x)) (u(v)X" (dy) a.c. B, u(x) = lim (25.18) (25.19) We will not follow the route laid out above, but use instead the Hardy-Little- wood maximal theorem (Theorem 25.17) to prove Theorem 25.20. The reason is mainly a didactic one, since this is a beautiful example of how weak-type maximal inequalities (i.e. inequalities like (25.14) and (25.11)) can be used to get a.e. convergence. More on this theme can be found in Krantz [26, pp. 27-30] and Garsia [18, pp. 1-4]. Our proof will also show that the limits in (25.18) and (25.19) can be strengthened to B{x}, where B is any ball containing x and, in the limit, shrinking to {x}.
Fantastic news! We've Found the answer you've been seeking!
Step by Step Answer:
Answer rating: 100% (3 reviews)
Equation 2519 As one would expect the derivative at x t...View the full answer

Answered By

PU Student
cost accounting
financial accounting
auditing
internal control
business analyst
tax
i have 3 years experience in field of management & auditing in different multinational firms. i also have 16 months experience as an accountant in different international firms. secondary school certification.
higher secondary school certification.
bachelors in mathematics.
cost & management accountant
4.80+
4+ Reviews
10+ Question Solved
Related Book For 

Question Posted:
Students also viewed these Business questions
-
Given that bar (n) runs in (logn) time, write a recurrence relation for the time taken by function foo (): void foo(int n) { if (n < 1) return; for (int i = 0; i < n; ++i) for (int j=0; j < n; ++j)...
-
KYC's stock price can go up by 15 percent every year, or down by 10 percent. Both outcomes are equally likely. The risk free rate is 5 percent, and the current stock price of KYC is 100. (a) Price a...
-
An industry consists of two firms with identical costs C(q) = 4+2q. The market demand is given by Q = 20- P, where P, Q stands for the market price and market demand respectively. Two firms compete...
-
Saeed does not keep proper books of account for his business but he has provided the following details of his assets and liabilities. Further information 1. Land and buildings have been revalued at...
-
The measured binding energy of KCI is 4.43eV. From the result of Problem 1, estimate the contribution to the binding energy of the repelling electron clouds at the equilibrium distance r0 = 0.28nm.
-
How many grams of N2 are needed to produce 2.17 mol of NH3 when reacted according to this chemical equation? N2(g) + 3H2(g) ( 2NH3(g)
-
Predicting elements in aluminum alloys. Aluminum scraps that are recycled into alloys are classified into three categories: soft-drink cans, pots and pans, and automobile crank chambers. A study of...
-
PepsiCo recently decided to invest more than $300 million for expansion in Brazil. Brazil offers considerable potential because it has 150 million people and their demand for soft drinks is...
-
At the beginning of the year, CCZ Corporation bought three used machines from Pequita Compression Incorporated. The machines Immediately were overhauled, Installed, and started operating. Because the...
-
Let \(f: \mathbb{R} ightarrow \mathbb{R}\) be a bounded increasing function. Show that \(f^{\prime}\) exists Lebesgue a.e. and \(f(b)--f(a) \geqslant \int_{(a, b)} f^{\prime}(x) d x\). When do we...
-
Use Theorem 24.6 to show that the martingale of Example 25.14 is uniformly integrable. Data from example 25.14 Data from theorem 24.6 Example 25.14 Consider in R" the half-open squares Qk (2):=z+[0,2...
-
Two parallel-plate vacuum capacitors have areas A1 and A2 and equal plate spacings d. Show that when the capacitors are connected in parallel, the equivalent capacitance is the same as for a single...
-
Country Analysis of Milan: -Discuss the overall cultural, political, legal, economic, and technological infrastructure issues that could potentially impact a product's introduction in Milan. -You...
-
Sarah is a highly introverted employee who works in a fast-paced sales team. Her manager recently noticed that Sarah tends to be reserved during team meetings and rarely volunteers her ideas....
-
Community Disaster Preparedness Review the information found at https://www.ready.gov/community-preparedness-toolkit Look at the steps involved in formulating community response to a disaster....
-
The topic of diversity, tolerance, and inclusion has been widely (and, in many cases, hotly) debated in society over the past several years. In my opinion (and this is up for discussion - hence this...
-
The objective of this coursework is for you to critically engage with the theory and practice of promoting wellbeing or equality, diversity and inclusion (including cultural diversity) for employees...
-
Steam enters a converging nozzle at 5.0 MPa and 400C with a negligible velocity, and it exits at 3.0 MPa. For a nozzle exit area of 60 cm2, determine the exit velocity, mass flow rate, and exit Mach...
-
The water in tank A is at 270 F with quality of 10% and mass 1 lbm. It is connected to a piston/cylinder holding constant pressure of 40 psia initially with 1 lbm water at 700 F. The valve is opened,...
-
Read Nonprofit Collaboration with Luxury Brands: Positive and Negative Effects for Cause-Related Marketing written by Boenigk and Schuchardt. (See Articles.) What precautions did the authors...
-
Should the law be changed to permit charitable nonprofits to endorse or oppose political candidates and provide financial support to political campaigns? Why or why not?
-
Do you think that nonprofits that receive government funds may feel inhibited from lobbying? If so, do you think such concerns are realistic or not?
-
How do warehouses and distribution centers differ? What is cross-docking and why might a company choose to cross-dock a product? What kinds of products can be delivered electronically? What kinds...
-
Strawberry Inc. has historically been an all-equity firm. The analyst expects EBIT to be $1.5B in perpetuity starting one year from now. The cost of equity for the company is 11.5% and the tax rate...
-
Guzman company received a 60- day, 5 % note for 54,000 dated July 12 from a customer on account. Determine the due date on note. Determine the maturity value of the note and journalize the entry of...

Study smarter with the SolutionInn App


