Lusin's theorem. The following steps furnish a proof of the following result.

Theorem (Lusin). Let \(\mu\) be an outer regular measure on the space \((X, d, \mathscr{B}(X))\). For every \(f \in \mathcal{L}^{p}(\mu), 1 \leqslant p0\) there is some \(\phi_{\epsilon} \in \mathcal{L}^{p}(\mu) \cap C_{b}(X)\), such that

\[\left\|\phi_{\epsilon}ight\|_{\infty} \leqslant\|f\|_{\infty} \leqslant \infty, \quad \mu\left\{f eq \phi_{\epsilon}ight\} \leqslant \epsilon \quad \text { and } \quad\left\|f-\phi_{\epsilon}ight\|_{p} \leqslant \epsilon\]

(i) Let \(A \in \mathscr{B}(X)\) such that \(\mathbb{1}_{A} \in \mathcal{L}^{p}(\mu)\). Then there is an open set \(U \supset A\) such that \(\mu(U)

(ii) Let \(f \in \mathcal{L}^{p}(\mu)\) such that \(0 \leqslant f \leqslant 1\). By the sombrero lemma (Corollary 8.9) there is a uniformly convergent sequence of simple functions which can be 'smoothed' using part (i).

(iii) Let \(f \in \mathcal{L}^{p}(\mu)\) such that \(c=\|f\|_{\infty}

(iv) Let \(f \in \mathcal{L}^{p}(\mu)\). Apply part (iii) to \(f_{R}:=(-R) \vee f \wedge R\).

Data from corollary 8.9

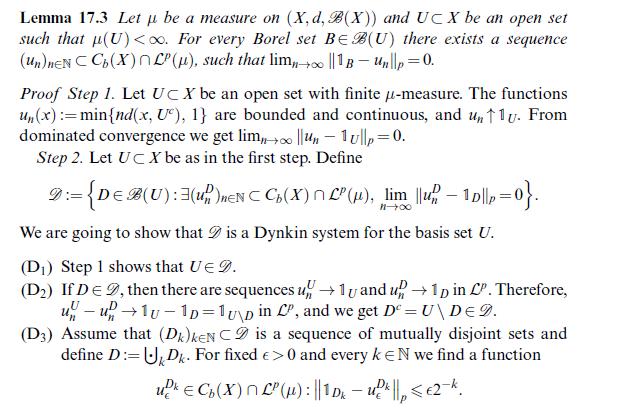
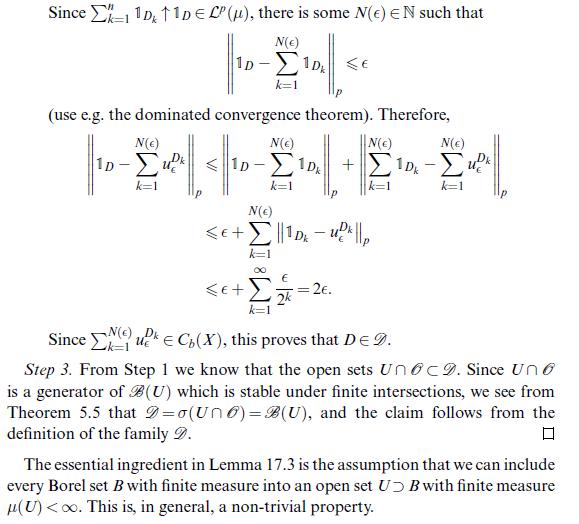
Data from lemma 17.3

Transcribed Image Text:

Corollary 8.9 Let (X, A) be a measurable space. Every A/B(R)-measurable function u: XR is the pointwise limit of simple functions fn = E(A) such that |fn| X} if X>0 if X < 0. {u+ >x} = X Since u = (-u)+, we have {ut > X} for all R. Thus ut are posi- tive measurable functions, and we can construct, as in Theorem 8.8, simple functions gut and hntu. Clearly, fn:=gn - hnut-u=uand |fn|=gn+hn c |fn(x) - u(x)