Show that every linear map \(f: \mathbb{R}^{n} ightarrow \mathbb{R}^{m}\) is \(\mathscr{B}\left(\mathbb{R}^{n}ight) / \mathscr{B}\left(\mathbb{R}^{m}ight)\)-measurable. Provide an example in which measurability of linear functions may fail if we use the completions of the Borel \(\sigma\)-algebras.

[ Think of suitable subsets of Borel null sets, see Problems 4.15 and 6.7.]

Data from problem 4.15

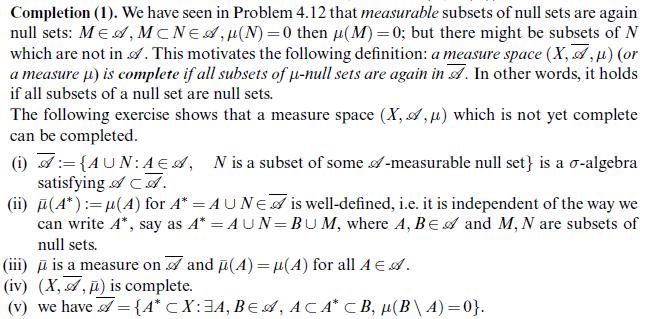
Data from problem 6.7

Completion (1). We have seen in Problem 4.12 that measurable subsets of null sets are again null sets: MEA, MCNEA, (N)=0 then (M)= 0; but there might be subsets of N which are not in . This motivates the following definition: a measure space (X, A, ) (or a measure ) is complete if all subsets of u-null sets are again in A. In other words, it holds if all subsets of a null set are null sets. The following exercise shows that a measure space (X, ,) which is not yet complete can be completed. (1) A:={AUN: AEA, N is a subset of some -measurable null set} is a -algebra satisfying CA. (ii) (4*):= (4) for A* = AUNE is well-defined, i.e. it is independent of the way we can write 4*, say as A* = AUN=BUM, where A, BEA and M, N are subsets of null sets. (iii) is a measure on and (4) = (A) for all A = A. (iv) (X, A,p) is complete. (v) we have = {A* CX: 3A, BEA, ACA* CB, (B\ 4)=0}.