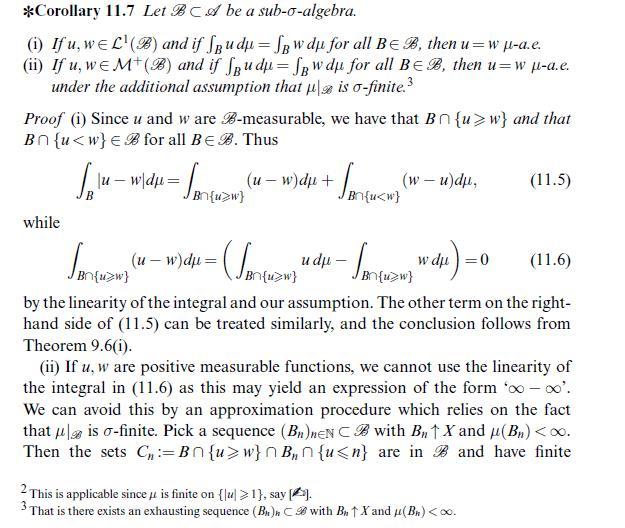
Show the following extension of Corollary 11.7 . Let \(\mathscr{C} \subset \mathscr{P}(X)\) be a \(\cap\)-stable generator of \(\mathscr{A}\) which contains a sequence \(C_{n} \uparrow X\) such that \(\mu\left(C_{n}ight)

\[\int_{C} u d \mu=\int_{C} w d \mu \quad \forall C \in \mathscr{C} \quad \Longleftrightarrow u=w \quad \mu \text {-a.e. }\]

[ use the uniqueness theorem for measures for \(C \mapsto \int_{C}\left(u^{ \pm}+w^{\mp}ight) d \mu\) and Corollary 11.7 .]

Data from corollary 11.7

Transcribed Image Text:

*Corollary 11.7 Let BCA be a sub-o-algebra. (i) Ifu, w L (B) and if feudu=fB w du for all B&B, then u=w -a.e. (ii) If u, w EM (B) and if fu du = fw du for all B&B, then u=w -a.e. under the additional assumption that l is o-finite. Proof (i) Since u and w are B-measurable, we have that Bn{u> w} and that Bn{u w} - + ( n{u