Find the natural frequency of the traffic sign system described in Problem 2.79 in torsional vibration about the \(z\)-axis by considering the masses of both the post and the sign.

The spring stiffness of the post in torsional vibration about the \(z\)-axis is given by \(k_{t}=\frac{\pi G}{2 l}\left(r_{0}^{4}-r_{i}^{4}\right)\). The mass moment of inertia of the sign about the \(z\)-axis is given by \(I_{0}=\frac{1}{12} m_{0}\left(d^{2}+b^{2}\right)\), where \(m_{0}\) is the mass of the sign.

Data From Problem 2.79:-

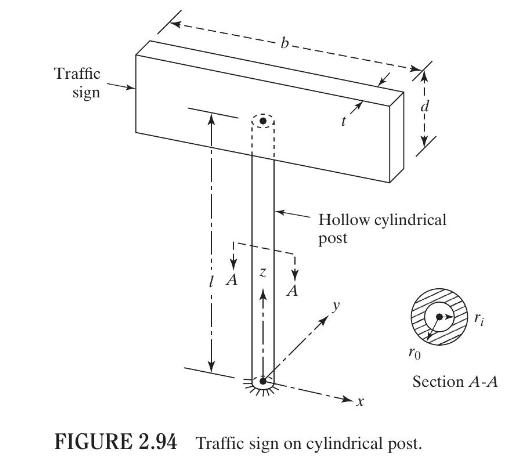
A steel hollow cylindrical post is welded to a steel rectangular traffic sign as shown in Fig. 2.94 with the following data:

Dimensions: \(l=2 \mathrm{~m}, r_{0}=0.050 \mathrm{~m}, r_{i}=0.045 \mathrm{~m}, b=0.75 \mathrm{~m}, d=0.40 \mathrm{~m}, t=0.005 \mathrm{~m}\); material properties: \(ho\) (specific weight) \(=76.50 \mathrm{kN} / \mathrm{m}^{3}, E=207 \mathrm{GPa}, G=79.3 \mathrm{GPa}\) Find the natural frequencies of the system in transverse vibration in the \(y z\) - and \(x z\)-planes by considering the masses of both the post and the sign.

Transcribed Image Text:

Traffic sign Hollow cylindrical post ro Section A-A FIGURE 2.94 Traffic sign on cylindrical post.