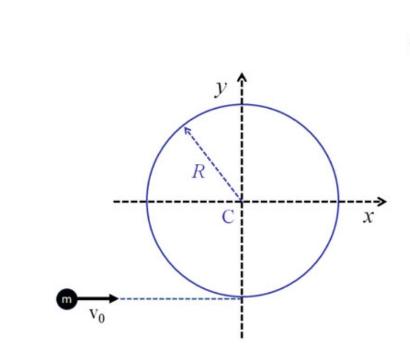
A projectile of mass \(m=2.5 \mathrm{~kg}\) is shot tangentially (see Fig.12.19) at the edge of a ring having radius \(R=50 \mathrm{~cm}\) and whose mass is equal (within measurement errors) to that of the projectile. The ring, free to move on a frictionless horizontal plane, is initially stationary. Before the collision the projectile's speed is \(v_{0}=3.0 \mathrm{~m} / \mathrm{s}\); after the collision the ring and the projectile remain together. Calculate, immediately after the collision:

1. the position of point \(\mathrm{D}\) representing the center of mass of the projectile+ring system and the distance \(d\) from the origin \(\mathrm{C}\) of the Cartesian reference frame;

2. the velocity \(\mathbf{v}_{D}\) of the center of mass of the system;

3. the angular speed \(\omega\) of the system;

4. the fraction of mechanical energy dissipated in the collision.

Fig. 12.19

Transcribed Image Text:

m Vo R y <