Question: A wave equation for light is where (phi) is a scalar potential. Show that the set of all linear transformations of the spacetime coordinates that

A wave equation for light is

10 + x2 2 + = 0.

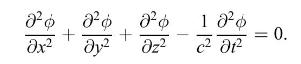
where $\phi$ is a scalar potential. Show that the set of all linear transformations of the spacetime coordinates that permit this wave equation to be written as we did, correspond to (i) four possible translations in space and time, (ii) three constant rotations of space, and (iii) three Lorentz transformations. Collectively, these are called the Poincaré transformations of spacetime.

10 + x2 2 + = 0.

Step by Step Solution

★★★★★

3.38 Rating (154 Votes )

There are 3 Steps involved in it

1 Expert Approved Answer

Step: 1 Unlock blur-text-image

Question Has Been Solved by an Expert!

Get step-by-step solutions from verified subject matter experts

Step: 2 Unlock

Step: 3 Unlock

Students Have Also Explored These Related Mechanics Questions!

If you dissolve 8.14 grams of calcium chloride, CaCl 2 , in enough water to make 125.0 mL of solution, what is the molarity of the solution?

ttth Suppose that the sequence of bags {Bn | n N} is recursively enumerated by the computable function e(n, x) = fn(x), [7 marks] Hence prove that the set of all recursive bags cannot be recursively...

re Regular Languages and Finite Automata (a) Let L be the set of all strings over the alphabet {a, b} that end in a and do not contain the substring bb. Describe a deterministic finite automaton...

\fCOMPLEX VARIABLES AND APPLICATIONS Eighth Edition James Ward Brown Professor of Mathematics The University of Michigan-Dearborn Ruel V. Churchill Late Professor of Mathematics The University of...

Need Help with Computational Physics HW for python on calculating electrostatic potential. Book from Computational physics by mark newman Need it solved in Overrelaxation method: Exercise 9.3: V 0...

1: GPS AMS 103, Spring 2017 Due: Thursday, February 9, 11:59 pm EST Quiz: Tuesday, February 14, in class Objectives To reinforce concepts relevant to GPS technology and the underlying mathematical...

Using "On the Spectrum of Hydrogen" by Niels Bohr -What is the main idea of the Author's argument? What is the main essence of the piece? -What ways does the author use to propose and support their...

Hi again, I was hoping you can help with this investment management assignment. I am attaching the word document. It is 4 problems about asset management. Thank you for your help! Fin 6310 1. Assume...

In the given question, two equations numbered I and Il are given. You have to solve both the equations and mark the appropriate answer I. 2a2 + 28a + 98 = 0 II. 9b2 - 2b - 7 = 0 1. a b 3....

4. Solve for x in log (3x-13x+28 +) = logs 0.5

The variable num 1 is the same as Num 1 in c + + Select one True False

1. Frank is 21 and has his first credit card with a monthly APR of 1.5%. He went on a shopping spree the first month and now has a balance of $1,000. Budget-wise, it would be nice if he could just...

To determine the equilibrium distribution $f(\sigma)$, we minimize the free en$\operatorname{ergy}(E-T S)$ of the system under the obvious constraint $\sum_{\sigma} f(\sigma)=1$. For this, we...

We shall consider only the Heisenberg model; the study of the Ising model is somewhat simpler. Following the procedure of Problem 12.7, we find that the "effective field" $\mathbf{H}_{a}$...

Congratulations! You've won a state lotto! The state lottery offers you the following (after-tax) payout options: B (Click the icon to view the payout options.) (Click the icon to view the present...

What does a retailer need to do to close the standards gap? Select one: A. A retailer needs to set service goals. O B. A retailer needs to commit to high-quality service. O C. A retailer needs to be...

Which of the following is a compound sentence I need to order a new auto sub dividing adjuster piece for the components behind it