Recall the symbol \(n(r)\), of equation (13.4.5), which denotes the number of closed graphs that can be drawn on a given lattice using exactly \(r\) bonds. Show that for a square lattice wrapped on a torus (which is equivalent to imposing periodic boundary conditions)

Data From Equation (13.4.5)

\[
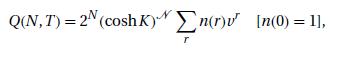
n(4)=N, \quad n(6)=2 N, \quad n(8)=\frac{1}{2} N^{2}+\frac{9}{2} N, \ldots
\]
Substituting these numbers into equation (13.4.5) and taking logs, one gets

Data From Equation (13.4.5)

\[
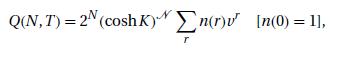
\ln Q(N, T)=N\left\{\ln \left(2 \cosh ^{2} K\right)+v^{4}+2 v^{6}+\frac{9}{2} v^{8}+\cdots\right\}, v=\tanh K
\]
Note that the term in \(N^{2}\) has disappeared - in fact, all higher powers of \(N\) do the same. Why?

Transcribed Image Text:

Q(N,T)=2(cosh K) n(r)" [n(0) = 1],