One does not have to be a biologist to appreciate the strong evolutionary advantage that natural selection

Question:

One does not have to be a biologist to appreciate the strong evolutionary advantage that natural selection confers on animals that can reduce their drag coefficients. It should be no surprise that the shapes and skins of many animals are highly streamlined. This is particularly true for aquatic animals. Of course, an animal minimizes drag while developing efficient propulsion and lift, which change the flow pattern. Comparisons of the properties of flows past different stationary (e.g., dead or towed) animals are, therefore, of limited value. The species must also organize its internal organs in 3 dimensions, which is an important constraint. The optimization varies from species to species and is suited to the environment. There are some impressive performers in the animal world.

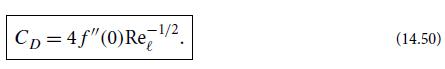
(a) First, idealize our animal as a thin rectangle with thickness t , length ℓ , and width w such that t « ℓ « w.⁵ Let the animal be aligned with the flow parallel to the ℓ direction. Assuming an area of A = 2ℓw, the drag coefficient is C_D = 1.33Re_ℓ^−0.5 [Eq. (14.50)]. This assumes that the flow is laminar. The corresponding result for a turbulent flow is C_D ≈ 0.072Re_ℓ^−0.2. Show that the drag can be considerably reduced if the transition to turbulence takes place at high Re_ℓ. Estimate the effective Reynolds number for an approximately flat fish like a flounder of size ∼0.3mthat can move with a speed∼0.3m s⁻¹, and then compute the drag force. Express your answer as a stopping length.

(b) One impressive performer is the mackerel (a highly streamlined fish), for which the reported drag coefficient is 0.0043 at Re ∼ 10⁵. Compare this with a thin plate and a sphere. (The drag coefficient for a sphere is C_D ∼ 0.5, assuming a reference area equal to the total area of the sphere. The drag decreases abruptly when there is a transition to turbulence, just as we found with the cylinder.)

(c) Another fine swimmer is the California sea lion, which has a drag coefficient of C_D = 0.0041 at Re ∼ 2 × 10⁶. How does this compare with a plate and a sphere?

Equation 14.50.