Suppose that some medium has a rest frame(unprimed frame) in which its energy flux and momentum density
Question:
Suppose that some medium has a rest frame(unprimed frame) in which its energy flux and momentum density vanish, T0j = Tj0 = 0. Suppose that the medium moves in the x direction with speed very small compared to light, v ≪ 1, as seen in a (primed) laboratory frame, and ignore factors of order v2.The ratio of the medium’s momentum density Gj´ = Tj´0´ (as measured in the laboratory frame) to its velocity vi = vδix is called its total inertial mass per unit volume and is denoted ρjiinert:
![]()
In other words, ρjiinert is the 3-dimensional tensor that gives the momentum density Gj´ when the medium’s small velocity is put into its second slot.(a) Using a Lorentz transformation from the medium’s (unprimed) rest frame to the (primed) laboratory frame, show that
![]()
(b) Give a physical explanation of the contribution Tjivi to the momentum density.
(c) Show that for a perfect fluid [Eq. (2.74b)] the inertial mass per unit volume is isotropic and has magnitude ρ + P, where ρ is the mass-energy density, and P is the pressure measured in the fluid’s rest frame:

See Ex. 2.26 for this inertial-mass role of ρ + P in the law of force balance.
Data from Ex. 2.26
Energy-Momentum Conservation for a Perfect Fluid(a) Derive the frame-independent expression (2.74b) for the perfect fluid stress energy tensor from its rest-frame components (2.74a).(b) Explain why the projection of ∇(vector) · T = 0 along the fluid 4-velocity, u(vector) . (∇(vector) · T ) = 0, should represent energy conservation as viewed by the fluid itself. Show that this equation reduces to
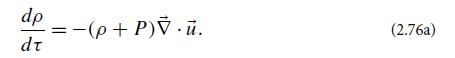
With the aid of Eq. (2.65), bring this into the form

where V is the 3-volume of some small fluid element as measured in the fluid’s local rest frame. What are the physical interpretations of the left- and right-hand sides of this equation, and how is it related to the first law of thermodynamics?
(c) Read the discussion in Ex. 2.10 about the tensor P = g + u(vector) ⊗ u(vector) that projects into the 3-space of the fluid’s rest frame. Explain why PμαTαβ;β = 0 should represent the law of force balance (momentum conservation) as seen by the fluid. Show that this equation reduces to
![]()
where a(vector) = du(vector)/dτ is the fluid’s 4-acceleration. This equation is a relativistic version of Newton’s F = ma. Explain the physical meanings of the left- and righthand sides. Infer that ρ + P must be the fluid’s inertial mass per unit volume. It is also the enthalpy per unit volume, including the contribution of rest mass.
![]()
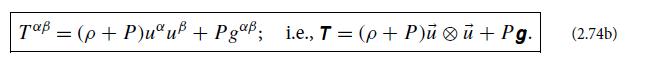
Step by Step Answer:

Modern Classical Physics Optics Fluids Plasmas Elasticity Relativity And Statistical Physics
ISBN: 9780691159027
1st Edition
Authors: Kip S. Thorne, Roger D. Blandford





