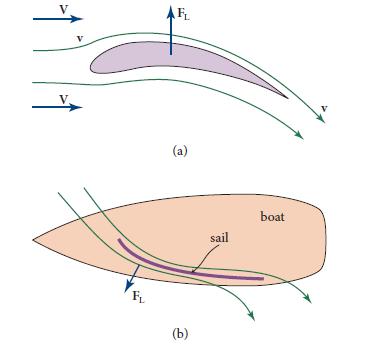
When an appropriately curved airfoil (e.g., an airplane wing) is introduced into a steady flow of air, the air has to flow faster along the upper surface than along the lower surface, which can create a lifting force (Fig. 14.7a). In this situation, compressibility and gravity are usually unimportant for the flow.

(a) Show that the pressure difference across the airfoil is given approximately by △P = 1/2ρ△(v²) = ρv△v. Hence show that the lift exerted by the air on an airfoil of length L is given approximately by

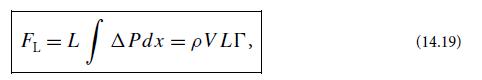
where Г is the circulation around the airfoil, and V is the air’s incoming speed in the airfoil’s rest frame. This is known as Kutta-Joukowski’s theorem. Interpret this result in terms of the conservation of linear momentum, and sketch the overall flow pattern.

(b) Explain why the circulation around an airplane wing (left orange curve in Fig. 14.2a) is the same as that around the wingtip’s vortex (right orange curve in Fig. 14.2a), and correspondingly explain why wingtip vortices are essential features of an airplane’s flight. Without them, an airplane could not take off.

(c) How might birds’ wingtip vortices (Fig. 14.2c) be related to the V-shaped configuration of birds in a flying flock?

(d) Explain how the same kind of lift as occurs on an airplane wing propels a sailboat forward when sailing upwind, as in Fig. 14.7b.

(e) Snakes, eels, and most fish undulate their bodies and/or fins as they swim. Draw pictures that explain how the same principle that propels a sailboat pushes these animals forward as well.

Figure 14.2.

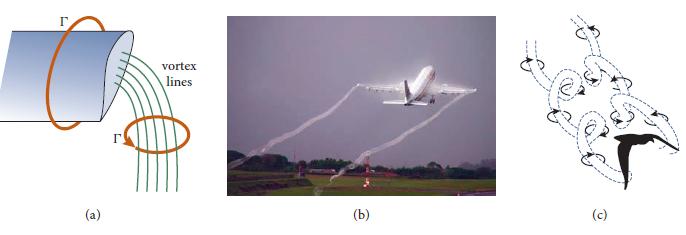
Figure 14.7.

Transcribed Image Text:

|F₁ = L = LS₁ APdx=pVLI, (14.19)