(a) A cylindrical solenoid occupies the interval −L/2 ≤ z ≤ L/2, has radius R, and is wound tightly with N turns of a wire which carries current I. Use superposition and derive an expression for the magnetic field on the symmetry axis in the form B₀(z) = (μ₀NI/L)f (z)ẑ. Compute the ratio B₀(±L/2)/B₀(0) in the limit when L << R.
(b) Fill the solenoid with a close-fitting rod of soft iron with effective susceptibility χ_m. Make the approximation that M(r) = M(z)ẑ, take account of the demagnetization field produced by the (magnetically) charged disks at either end of the rod, but ignore the effect of volume magnetic charge. Show that, inside the solenoid,

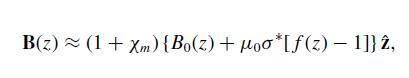
where (when L >> R)

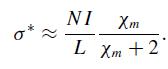
(c) Calculate the amplification of the magnetic field produced by the soft iron at z = 0 and at z = ± L/2. Comment on the result when χ_m >> 1.

Transcribed Image Text:

B(z) (1 + Xm) {Bo(z) + Moo*[ƒ(z) - 1]} 2,