Let B(x, z) be the magnetic field produced by a surface current density K(y, z) = K(z)

Question:

Let B(x, z) be the magnetic field produced by a surface current density K(y, z) = K(z) ŷ confined to the x = x₀ plane.

(a) Show that the Biot-Savart law for this situation reduces to a one dimensional convolution integral for each component of B.
(b) Confine your attention to x < x₀and show that

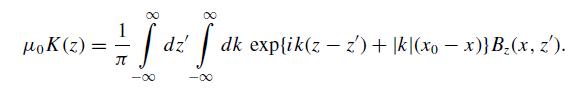
(c) Why does the single component Bz(x, z) evaluated at one (arbitrary) value of x < x₀ provide enough information to determine j (z)?

Fantastic news! We've Found the answer you've been seeking!

Step by Step Answer:

Related Book For book-img-for-question

Modern Electrodynamics

ISBN: 9780521896979

1st Edition

Authors: Andrew Zangwill

See More Books

Question Posted: Mar 29, 2023 01:41 AM

See More Questions

A long, straight wire carrying a current of 200 A, runs trough a cubical wooden box, entering and leaving through holes in the centres of opposite faces (Fig). The length of each side of the e- box...
This problem leads you through a study of electric and magnetic fields as viewed by two different observers, that is, in two different reference frames. Suppose a charge q 1 is moving with a constant...
A power line carries a current of 95 A along the tops of 8.5-m-high poles. What is the magnitude of the magnetic field produced by this wire at the ground? How does this compare with the Earths field...
Let x be the number of magazines a person reads every week. Based on a sample survey of adults, the following probability distribution table was prepared. Find the mean and standard deviation of x. 0...
Draw the distribution that should result from an honest roulette wheel. Draw the distribution from a dishonest roulette wheel. Assuming samples of 500 spins were taken from each wheel, what would the...
Why do you think the striking public school teachers in all five states successfully obtained higher wages and better funding for their schools?
Martin Alexander created a pilot script for a new television sitcom entitled Loony Ben. Beginning in March 2006, Alexander submitted copies of the script to agents, producers, directors, production...
Presented below are the purchases and cash payments journals for Fornelli Co. for its first month of operations. In addition, the following transactions have not been journalized for July. The cost...
84. Which is incorrect order of -I (1) F>Cl> Br>I (2) NR,>-NH3>-NO (3) OCH3>-OH>NH, > -C = CH>H (4) 85. Which of the following compounds is most basic? (1) CH3NH2 (3) CH,-N-CH3 CH (2) CH3-NH-CH3 (4)...
The figure below shows an infinitely long current filament wound in the form of a circular helix with radius R and pitch l , i.e., is the distance along the z-axis occupied by one wind of the helix....
Use the solid angle representation of the magnetic scalar potential (r) to find B(r) everywhere for an infinite, straight line of current I . State carefully the surface you have chosen to cut the...
A hollow sphere of radius 0.15 m, with rotational inertia 1 = 0.040 kg- m 2 about a line through its center of mass, rolls without slipping up a surface inclined at 30 to the horizontal. At a certain...
The CEO's compensation contract includes a provision for a bonus of 15% of income before the bonus but after income taxes (30% rate). Income before bonus and income tax is $1.2 million for the...
Q8: resample the Order_Date into quarters and determine the quarter with the highest profitability from 2014 to 2017. (2 points) hint: check resample() documentation and resample the date to 3 months...
2. Develop a temperature converter GUI JavaFX application that consists of two labels and two text fields. Typing a temperature into the Celsius field and then pressing the "Enter" key causes the...
On February 1, a US company buys goods from a UK company for 80,000 British pounds, payable on March 1. The exchange rate on February 11s 0.752 per US dollar, and the exchange rate on March 1 is...
Answer the following prompt questions for this discussion with your fellow students: 1. What kinds of stories, topics, or issues are not being covered well by mainstream newspapers (in print or...
We suggested in the text that there may be technological reasons for the barriers to entry required for the existence of monopoly. In this exercise, we consider two examples. A: Microsoft and your...
Write an SQL statement to display all data on products having a QuantityOnHand greater than 0.
Suppose it is known that the graph of the function y = ax 3 + bx 2 + cx + d passes through four given points (x i , y i ), i = 1, 2, 3, 4. Write a userdefined function that accepts these four points...
Create an anonymous function for 10e -2x and use it to plot the function over the range 0 x 2.
Create an anonymous function for 20x 2 - 200x + 3 and use it a. To plot the function to determine the approximate location of its minimum b. With the fminbnd function to precisely determine the...
(Computing rates of return) From the following price data, compute the annual rates of return for Asman and Salinas. Time 1 Asman $11 Salinas $30 2 3 4 12 10 14 29 32 34 (Click on the icon in order...
A firm is considering investing in a project with the following cash flows: Year Net cash 1 2 3 4 5 6 7 8 2,000 3,000 4,000 3,500 3,000 2,000 1,000 1,000 flow ($) The project requires an initial...
Share a situation in which you have actively (or passively) resisted organizational control efforts. Describe your motivation in this situation. What do you think should be the limits of the degree...

Let B(x, z) be the magnetic field produced by a surface current density K(y, z) = K(z)

Question:

μοΚ (2) - ² Ĵ az Ĵ dk dz' = 88 -∞ f dk exp{ik(zz) + k|(xox)} B₂(x, z).

Step by Step Answer:

a b Define the Fourier transform pairs c Br 440 Kx rr r r ...View the full answer

Modern Electrodynamics

Students also viewed these Engineering questions