Let the origin of coordinates be centered on a compact, time-harmonic source of electromagnetic radiation. The time-averaged power radiated into a differential element of solid angle d Ω centered on an observation point r has the form

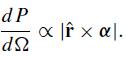
The vector ∝  = p₀ if the source has a time-dependent electric dipole moment p(t) = p₀ cos ωt. The vector α = m₀ × r̂ if the source has a time-dependent magnetic dipole momentum(t) = m₀ cos ωt. For this problem, consider a source where p(t) and m(t) are present simultaneously.
(a) Show that the time-averaged angular distribution of power generally exhibits interference between the two types of dipole radiation. Under what conditions is there no interference?
(b) Show that the time-averaged total power emitted by the source does not exhibit interference.

dP alixal.