What magnetization M(r) imposed on an infinite piece of iron produces the largest magnetic field at a given point? Francis Bitter (a pioneer in the design of high-field magnets) posed this problem in 1936. Since the magnetization of a ferromagnet is produced by electron spins, we need first to find the direction of a point magnetic dipole with moment m located at r = (r, θ, φ) that maximizes the z-component of its magnetic field at the origin.

(a) Show that the maximum of B_z(0) occurs when 2 tan ∝ = tan θ, where r̂ · m̂ = cos ∝ and r lies between m and ẑ in the plane φ = 0.
(b) Show that the maximal condition can be written 1 / 2 tan θ = rdθ/dr. Use this to establish that the locus of desired spin directions in the ferromagnet is indistinguishable from the field lines of a suitably oriented point magnetic dipole at the origin.
(c) Show that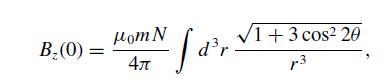where N is the number of spins/volume. Perform the integral for the case of a spherical shell of iron with inner radius r₁ and outer radius r₂.

Transcribed Image Text:

B₂(0)= Mom N 4л √1+3 cos² 20 r3 ·fd³r.