Show that by making the substitution Show that the solution of this equation is v = 1

Question:

Show that by making the substitution

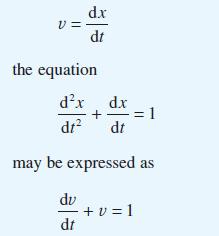
V = d.x dt the equation dx dt ap + dx dt may be expressed as = 1 - + v = 1 dt

Show that the solution of this equation is v = 1 + Ce^–t and hence find x(t). This technique is a standard method for solving second-order differential equations in which the dependent variable itself does not appear explicitly. Apply the same method to obtain the solutions of the differential equations

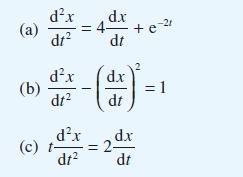
dx d.x (a) = 4 dt dt (b) dx dt dx (c) t- d.x dt +e-1 dt dt el = 2dx = 1