Follow these steps to show that solutions to Kummer's Equation (3.7.46) can be written in terms of

Question:

Follow these steps to show that solutions to Kummer's Equation (3.7.46) can be written in terms of Laguerre polynomials Ln (x ), which are defined according to a generating function as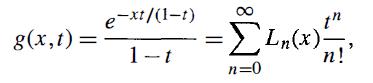

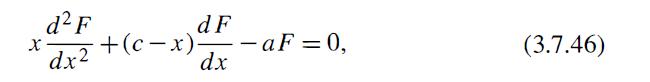
where 0

(a) Prove that L_n(0) = n ! and L₀(x) = 1.

(b) Differentiate g(x , t) with respect to x, show that

and find the first few Laguerre polynomials.

(d) Now show that Kummer's Equation is solved by deriving

and associate n with the principal quantum number for the hydrogen atom.

Fantastic news! We've Found the answer you've been seeking!

Step by Step Answer:

Related Book For book-img-for-question

Modern Quantum Mechanics

ISBN: 9780805382914

2nd Edition

Authors: J. J. Sakurai, Jim J. Napolitano

See More Books

Question Posted: Aug 05, 2023 07:45 AM

See More Questions

find one example from the news media that you can link --either videos, podcasts, articles, reports, television broadcasts, etc.--that exhibit what you take to be a valid and sound deductive argument.
According to Zeno's paradox any object in motion must arrive at the halfway point before it can arrive at its destination. Once arriving at the halfway point, the remaining distance is once again...
How do you reconcile the need to treat much of the information in the plan as company confidential information, yet at the same time ensure that the plan is readily available when an attack occurs?
Carlberg Company has two manufacturing departments, Assembly and Painting. The Assembly department started 11,000 units during November. The following production activity in both units and costs...
Rock-Paper-Scissors is a simple game in which two players compete by simultaneously choosing rock, paper, or scissors. According to the classic rules of the game, paper beats rock, scissors beats...
Use the model of perfect competition described in this chapter to explain, illustrate, or elaborate on the following statements. a. "Increasing competition from new firms entering the market is good...
Explain the difference between a producer and a consumer.
Data for Cheng Inc. are presented in P17-9A. Further analysis reveals that accounts payable pertain to merchandise creditors. In p17-9A, Condensed financial data of Cheng Inc. follow. Additional...
Round 1: Buhi wants to renew interests in its OG Bag line and identify the optimal target audiences for OG products by using SMM. Your advertising budget is $500. Your assignment will be to review...
Show that the orbital angular-momentum operator L commutes with both the operators p 2 and x 2 ; that is, prove (3.7.2). [L,p] = [L,x] = 0 (3.7.2)
Let U = e iG 3 e iG2 e iG3 , where (a ,, ) are the Eulerian angles. In order that U represent a rotation (a ,, ), what are the commutation rules that must be satisfied by the G k ? Relate G to the...
Examine the following adjusting entries and determine which ones should be reversed. Show the reversing entries that should be recorded in the general journal as of January 1, 2020. Include...
On January 1, Year 8, Fazli Co. acquired all of the common shares of Gervais. Both companies had no inventory on hand at the beginning of the year. The following transactions occurred in January and...
A life insurance company issues a 10-year term assurance policy to a life aged [50], with sum insured 100,000. Level premiums are paid monthly in advance throughout the term. The interest rate is 5%...
The volume of a cone is given by the equation V = Abase h, where Abase is the area of the circular base with radius r and h is the height of the cone, as shown in the diagram. What is the volume of a...
Answer the question on the basis of the following cost data. Average Fixed Average Variable Output Cost Cost 1 $50.00 $100.00 2 25.00 80.00 3 16.67 66.67 4 12.50 65.00 5 10.00 68.00 6 8.37 73.33 7...
On July 9, you purchased 800 shares of Blue Water stock for $32 a share. On August 4, you sold 200 shares of this stock for $33 a share. You sold an additional 200 shares on August 14 at a price of...
Health researchers at a large HMO base their cost predictions for measles on the belief that 98% of children have been vaccinated against measles. A random survey of medical records at many schools...
Air pollution generated by a steel mill is an example of a) a positive production externality. b) a negative production externality. c) a public good. d) the free-rider problem. State and local taxes...
Write the K b reaction of CN - . Given that the K a value for HCN is 6.2 10 -10 , calculate K b for CN - .
Write the K a2 reaction of phosphoric acid (H 3 PO 4 ) and the K b2 reaction of disodium oxalate (Na 2 C2O 4 ).
(a) Using only K sp from Table 6-3, calculate how many moles of Ca(OH) 2 will dissolve in 1.00 L of water. (b) How will the solubility calculated in part (a) be affected by the K 1 reaction in Table...
What is the velocity difference between the perihelion velocity at 1 AU (semimajor axis=2AU) and the circular orbit velocity at 1 AU?
When a nonlinear (or unstable) load is connected to a three phase motor system, the harmonic currents in the neutral wire will become very high? Why or why not ?
Assume that a 20.0 volt energy source is in a circuit that has a resistance of 4.00 ohms. Determine the power consumption in watts.

Follow these steps to show that solutions to Kummer's Equation (3.7.46) can be written in terms of

Question:

g(x,t) = e-xt/(1-t) 1-1 = Στ()*, n=0 n!

Step by Step Answer:

To answer this question lets work through each part step by step using the information given and the definitions provided a Prove that Ln0 n and L0x 1 First lets consider the generating function for t...View the full answer

Modern Quantum Mechanics

Students also viewed these Engineering questions